AI tutor
Full solution
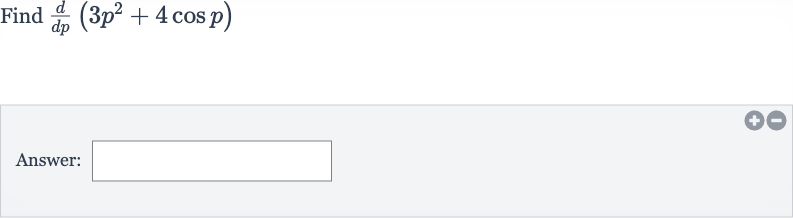
Q. Find Answer:
- Differentiate : Differentiate the term with respect to . To differentiate , we use the power rule, which states that the derivative of with respect to is . Therefore, the derivative of is .
- Differentiate : Differentiate the term with respect to . To differentiate , we use the chain rule and the fact that the derivative of with respect to is . Therefore, the derivative of is .
- Combine derivatives: Combine the derivatives from Step and Step .The derivative of the entire function with respect to is the sum of the derivatives of its terms, which is .
More problems from Find trigonometric functions using a calculator
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
Question
. Find the value of in degrees.Write your answer in simplified, rationalized form. Do not round.____
Get tutor helpQuestionGet tutor help