Full solution
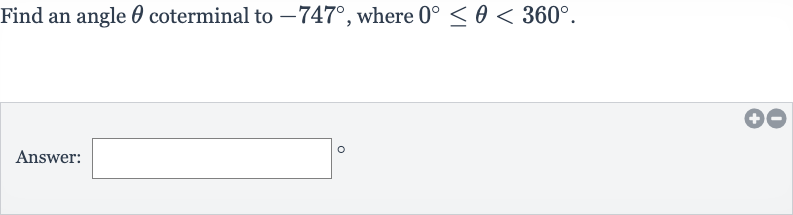
Q. Find an angle coterminal to , where .Answer:
- Add °: To find a coterminal angle, we can add or subtract multiples of to the given angle until the result is within the desired range of to . Since is negative, we will add repeatedly until we get a positive angle in the specified range.
- Add °: First, add ° to : . The result is still negative, so we need to add ° again.
- Add °: Add ° to : . The result is still negative, so we need to add ° one more time.
- Add °: Add ° to : . The result is now positive and within the range of to , so we have found a coterminal angle.
More problems from Find trigonometric ratios using reference angles
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
Question
. Find the value of in degrees.Write your answer in simplified, rationalized form. Do not round.____
Get tutor helpQuestionGet tutor help