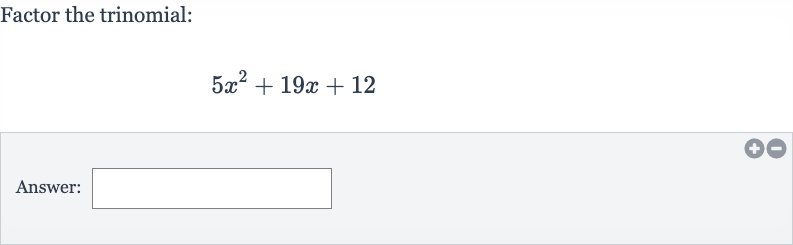
Factor the trinomial: 5x^(2)+19 x+12 Answer:

Question

Factor the trinomial:  5x^(2)+19 x+12 Answer:

Bytelearn · Accepted Answer

Identify Coefficients: Identify the coefficients of the trinomial. The trinomial is in the form ax^2 + bx + c, where a = 5, b = 19, and c = 12.Find Multiplying Numbers: Find two numbers that multiply to ac (5 * 12 = 60) and add up to b (19). We need to find two numbers that multiply to 60 and add up to 19. After checking possible pairs of factors of 60, we find that 4 and 15 satisfy these conditions. 4 * 15 = 60 4 + 15 = 19Rewrite Middle Term: Rewrite the middle term (19x) using the two numbers found (4 and 15). The trinomial 5x^2 + 19x + 12 can be expressed as 5x^2 + 4x + 15x + 12.Factor by Grouping: Factor by grouping. Group the terms into two pairs: (5x^2 + 4x) and (15x + 12). Factor out the greatest common factor from each pair. From the first pair, we can factor out x, resulting in x(5x + 4). From the second pair, we can factor out 3, resulting in 3(5x + 4).Write Factored Form: Write the factored form by combining the common factors. Since both groups contain the factor (5x + 4), we can combine them to get (x + 3)(5x + 4) as the factored form of the trinomial.

Factor the trinomial: $\newline$ $5 x^{2}+19 x+12$ $\newline$ Answer:

Full solution

More problems from Factor polynomials

Related topics

Factor the trinomial:\newline5x2+19x+12 5 x^{2}+19 x+12 5x2+19x+12\newlineAnswer:

Factor the trinomial: $\newline$ $5 x^{2}+19 x+12$ $\newline$ Answer: