AI tutor
Full solution
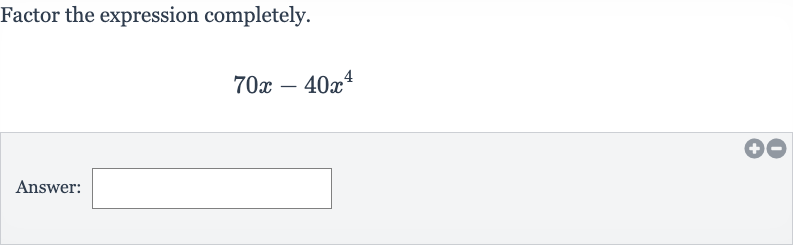
Q. Factor the expression completely.Answer:
- Identify GCF: Identify the greatest common factor (GCF) of the terms in the expression.The terms are and . The GCF of the numerical coefficients ( and ) is . Both terms also have an term, so the GCF is .
- Factor out GCF: Factor out the GCF from the expression.We can write the expression as .
- Check for further factoring: Check if the remaining expression inside the parentheses can be factored further.The expression inside the parentheses is , which is a difference of two cubes since is and is .
- Factor difference of cubes: Factor the difference of two cubes using the formula . Here, is and is , so we get .
- Simplify factored form: Simplify the factored form of the difference of two cubes.We get .
- Combine with GCF: Combine the GCF factored out earlier with the factored form of the difference of two cubes.The completely factored expression is .
- Check for further simplification: Check for any further simplification or common factors in the factored terms.There are no further common factors, and the expression is fully simplified.
More problems from Evaluate integers raised to rational exponents
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help