AI tutor
Full solution
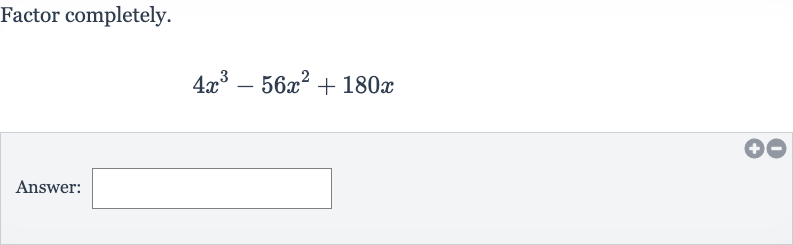
Q. Factor completely.Answer:
- Identify GCF of terms: Identify the greatest common factor (GCF) of the terms in the polynomial . The GCF of , , and is , since is the largest factor that divides evenly into all three terms.
- Factor out GCF: Factor out the GCF from each term in the polynomial.So, factoring out gives us .
- Factor quadratic expression: Factor the quadratic expression . We need to find two numbers that multiply to and add up to . The numbers that satisfy these conditions are and . So, can be factored as .
- Write fully factored form: Write the fully factored form of the original polynomial.Since we factored out in Step and factored the quadratic in Step , the fully factored form of the polynomial is .