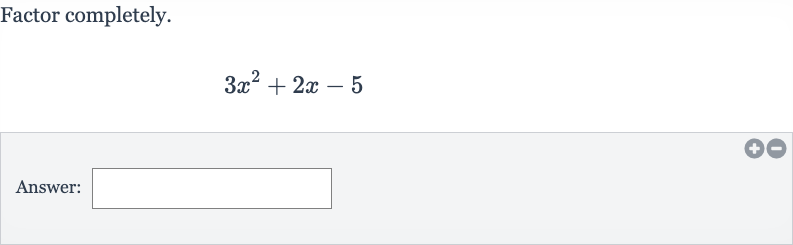
Factor completely. 3x^(2)+2x-5 Answer:

Question

Factor completely.  3x^(2)+2x-5 Answer:

Bytelearn · Accepted Answer

Identify Coefficients: Identify the coefficients of the quadratic expression. The quadratic expression is 3x^2 + 2x - 5. Here, a = 3, b = 2, and c = -5.Check Factorability: Determine if the quadratic expression can be factored using simple factoring techniques. Since ac = (3)(-5) = -15, we need to find two numbers that multiply to -15 and add up to the middle coefficient, b = 2.Find Suitable Numbers: Find two numbers that meet the criteria. The numbers 5 and -3 multiply to -15 and add up to 2. 5 * -3 = -15 5 + (-3) = 2Rewrite Middle Term: Write the middle term as a sum of two terms using the numbers found. The expression 3x^2 + 2x - 5 can be rewritten as 3x^2 + 5x - 3x - 5.Factor by Grouping: Factor by grouping. Group the terms as (3x^2 + 5x) + (-3x - 5). Factor out the greatest common factor from each group. The first group has a common factor of x, and the second group has a common factor of -1. This gives us x(3x + 5) - 1(3x + 5).Factor Common Binomial: Factor out the common binomial factor. The common binomial factor is (3x + 5). The factored form is (x - 1)(3x + 5).

Factor completely. $\newline$ $3 x^{2}+2 x-5$ $\newline$ Answer:

Full solution

More problems from Factor polynomials

Related topics

Factor completely.\newline3x2+2x−5 3 x^{2}+2 x-5 3x2+2x−5\newlineAnswer:

Factor completely. $\newline$ $3 x^{2}+2 x-5$ $\newline$ Answer: