Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

Factor completely.

3x^(2)+19 x-14
Answer:

Factor completely. $\newline$ $3 x^{2}+19 x-14$ $\newline$ Answer:

View step-by-step help

View step-by-step help

Factor quadratics

Full solution

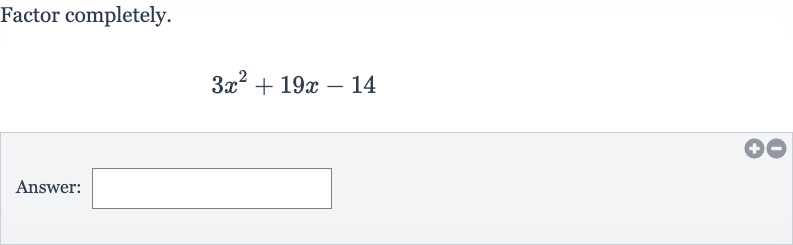
Q. Factor completely.

\newline

3 x^{2}+19 x-14

\newline

Answer:

Identify Quadratic Trinomial: Identify the quadratic trinomial. $\newline$ The given expression is $3x^2 + 19x - 14$ , which is a quadratic trinomial of the form $ax^2 + bx + c$ .
Find Multiplying Numbers: Look for two numbers that multiply to $ac$ (product of the coefficient of $x^2$ and the constant term) and add to $b$ (the coefficient of $x$ ). $\newline$ In this case, $ac = 3 \times (-14) = -42$ and $b = 19$ . $\newline$ We need to find two numbers that multiply to $-42$ and add up to $19$ .
Determine Two Numbers: Find the two numbers. $\newline$ After checking possible factors of $-42$ , we find that $21$ and $-2$ multiply to $-42$ and add up to $19$ .
Rewrite Middle Term: Rewrite the middle term using the two numbers found in Step $3$ . $\newline$ We can express $19x$ as $21x - 2x$ , so the expression becomes: $\newline$ $3x^2 + 21x - 2x - 14$ .
Factor by Grouping: Factor by grouping. $\newline$ Group the terms into two pairs: $(3x^2 + 21x)$ and $(-2x - 14)$ . $\newline$ Factor out the greatest common factor from each pair: $\newline$ $3x(x + 7) - 2(x + 7)$ .
Factor Common Binomial: Factor out the common binomial factor. $\newline$ The common binomial factor is $(x + 7)$ , so we factor it out: $\newline$ $(3x - 2)(x + 7)$ .

More problems from Factor quadratics

Question

Factor out the greatest common factor. If the greatest common factor is

1

, just retype the polynomial.

\newline

4x^3 - 6x^2

\newline

Posted 3 months ago

Question

x^4 + 8x^2 + 16

\newline

All factors in your answer should have integer coefficients.

\newline

Posted 3 months ago

Question

Find the binomial that completes the factorization.

\newline

t^3 + u^3 = (\underline{\hspace{1cm}}) (t^2 - tu + u^2)

Posted 3 months ago

Question

\newline

g^2 - 11g + 18

Posted 3 months ago

Question

\newline

2y^3 - y^2 + 16y - 8

\newline

\newline

Posted 3 months ago

Question

\newline

2x^8 + 7x^2

\newline

Posted 4 months ago

Question

\newline

9y^{12} + 4y^5 - y^2

\newline

Posted 4 months ago

Question

\newline

6a^4 + 3a^3 - a + 2

\newline

Posted 4 months ago

Question

\newline

4c^7 - 2c^5 + c^2 - 5

\newline

Posted 4 months ago

Question

\newline

7m^9 + 2m^6 - m^3 + 4m - 10

\newline

Posted 4 months ago