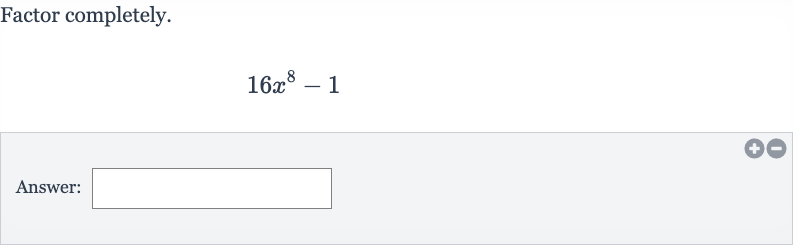
Factor completely. 16x^(8)-1 Answer:

Question

Factor completely.  16x^(8)-1 Answer:

Bytelearn · Accepted Answer

Determine Approach: Determine the approach to factor 16x^8 - 1. This expression is a difference of squares because it can be written as (4x^4)^2 - 1^2.Apply Formula: Apply the difference of squares formula. The difference of squares formula is a^2 - b^2 = (a - b)(a + b). Here, a = 4x^4 and b = 1. So, 16x^8 - 1 = (4x^4)^2 - 1^2 = (4x^4 - 1)(4x^4 + 1).Check Further Factoring: Check if further factoring is possible. The term 4x^4 + 1 cannot be factored further as it is not a difference of squares. However, 4x^4 - 1 is a difference of squares since it can be written as (2x^2)^2 - 1^2.Factor Further: Factor 4x^4 - 1 further using the difference of squares formula. We have (2x^2)^2 - 1^2 = (2x^2 - 1)(2x^2 + 1).Check Factoring: Check if further factoring is possible for the new terms. The term 2x^2 + 1 cannot be factored further as it is not a difference of squares. The term 2x^2 - 1 is also a difference of squares since it can be written as (x√2)^2 - 1^2.Factor Further: Factor 2x^2 - 1 further using the difference of squares formula. We have (x√2)^2 - 1^2 = (x√2 - 1)(x√2 + 1).Combine Factored Terms: Combine all the factored terms to get the final factored form. The fully factored form of 16x^8 - 1 is (4x^4 + 1)(2x^2 - 1)(2x^2 + 1) = (4x^4 + 1)(x√2 - 1)(x√2 + 1).

Factor completely. $\newline$ $16 x^{8}-1$ $\newline$ Answer:

Full solution

More problems from Factor quadratics: special cases

Related topics

Factor completely.\newline16x8−1 16 x^{8}-1 16x8−1\newlineAnswer:

Factor completely. $\newline$ $16 x^{8}-1$ $\newline$ Answer: