AI tutor
Full solution
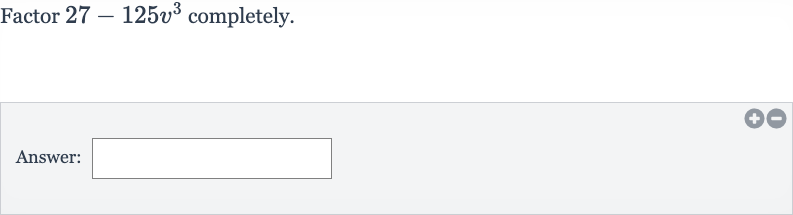
Q. Factor completely.Answer:
- Identify Type of Factoring: Identify the type of factoring required for . This is a difference of cubes since both terms are perfect cubes: and .
- Write Down Formula: Write down the formula for factoring a difference of cubes.The formula for factoring a difference of cubes is .
- Apply Formula to : Apply the formula to .Let and , then we have:
- Calculate Squares and Product: Calculate the squares and the product of and .
- Write Final Factored Form: Write the final factored form.The factored form of is .