Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

Express the given expression without logs, in simplest form. Assume all variables represent positive values.

(e^(-2ln 12sqrty))
Answer:

Express the given expression without logs, in simplest form. Assume all variables represent positive values. $\newline$ $\left(e^{-2 \ln 12 \sqrt{y}}\right)$ $\newline$ Answer:

View step-by-step help

View step-by-step help

Full solution

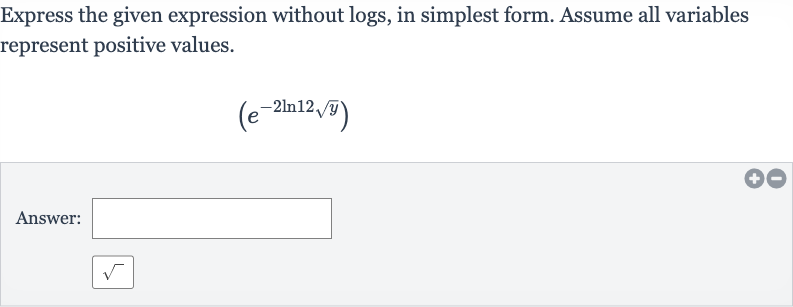
Q. Express the given expression without logs, in simplest form. Assume all variables represent positive values.

\newline

\left(e^{-2 \ln 12 \sqrt{y}}\right)

\newline

Answer:

Apply Power Rule: We are given the expression $e^{-2\ln(12\sqrt{y})}$ . To simplify this expression, we will use the property of logarithms that states $e^{\ln(x)} = x$ . This property allows us to remove the natural logarithm when it is the exponent of $e$ .
Apply Property of e: First, we need to apply the power rule of logarithms, which states that $\ln(a^b) = b\cdot\ln(a)$ . In our case, we have $-2\ln(12\sqrt{y})$ , which can be written as $\ln((12\sqrt{y})^{-2})$ .
Simplify Expression: Now, we can apply the property $e^{\ln(x)} = x$ to our expression. This means that $e^{\ln((12\sqrt{y})^{-2})}$ simplifies to $(12\sqrt{y})^{-2}$ .
Apply Negative Exponent Rule: Next, we need to simplify $(12\sqrt{y})^{-2}$ . This is the same as $(12y^{1/2})^{-2}$ , which can be simplified by applying the negative exponent rule, which states that $a^{-n} = 1/a^n$ .
Square Coefficient and Term: Applying the negative exponent rule, we get $\frac{1}{(12y^{1/2})^2}$ . Now we need to square both the coefficient $12$ and the term $y^{1/2}$ .
Square Coefficient and Term: Applying the negative exponent rule, we get $\frac{1}{(12y^{\frac{1}{2}})^2}$ . Now we need to square both the coefficient $12$ and the term $y^{\frac{1}{2}}$ . Squaring $12$ gives us $144$ , and squaring $y^{\frac{1}{2}}$ gives us $y^{\frac{1}{2} \times 2} = y^1 = y$ . So, our expression becomes $\frac{1}{144y}$ .

More problems from Power rule

Question

\newline

81^{\frac{1}{2}}

\newline

Posted 9 months ago

Question

\newline

(-32)^{1/5}

\newline

Posted 9 months ago

Question

Simplify. Assume all variables are positive.

\newline

\frac{w^{\frac{4}{3}}}{w^{\frac{7}{3}}}

\newline

Write your answer in the form

A

\frac{A}{B}

A

B

are constants or variable expressions that have no variables in common. All exponents in your answer should be positive.

\newline

Posted 5 months ago

Question

Simplify. Assume all variables are positive.

\newline

(2r^{\frac{1}{3}})^8

\newline

\newline

Write your answer in the form

A

\frac{A}{B}

A

B

are constants or variable expressions that have no variables in common. All exponents in your answer should be positive.

\newline

Posted 5 months ago

Question

Simplify. Assume all variables are positive.

\newline

v^{\frac{7}{4}} \cdot v^{\frac{9}{4}}

\newline

\newline

Write your answer in the form

A

\frac{A}{B}

A

B

are constants or variable expressions that have no variables in common. All exponents in your answer should be positive.

\newline

\newline

Posted 5 months ago

Question

\newline

1^{\frac{1}{4}} \cdot 1^{\frac{1}{4}}

\newline

Posted 5 months ago

Question

\newline

32^{(-1/5)}

Posted 7 months ago

Question

Simplify. Assume all variables are positive.

\newline

\frac{w^{\frac{3}{2}}}{w^{\frac{5}{2}}}

\newline

\newline

Write your answer in the form

A

\frac{A}{B}

A

B

are constants or variable expressions that have no variables in common. All exponents in your answer should be positive.

\newline

\newline

Posted 9 months ago

Question

Simplify. Assume all variables are positive.

\newline

(27x)^{\frac{2}{3}}

\newline

\newline

Write your answer in the form

A

\frac{A}{B}

A

B

are constants or variable expressions that have no variables in common. All exponents in your answer should be positive.

\newline

Posted 8 months ago

Question

Simplify. Assume all variables are positive.

\newline

\frac{x^{\frac{1}{4}}}{x^{\frac{11}{4}}}

\newline

\newline

Write your answer in the form

A

\frac{A}{B}

A

B

are constants or variable expressions that have no variables in common. All exponents in your answer should be positive.

\newline

\newline

Posted 7 months ago

Related topics

Algebra - Order of Operations Algebra - Distributive Property `X` and `Y` Axes Geometry - Scalene Triangle Common Multiple Geometry - Quadrant