Resources

Testimonials

Plans

Resources

Testimonials

Plans

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

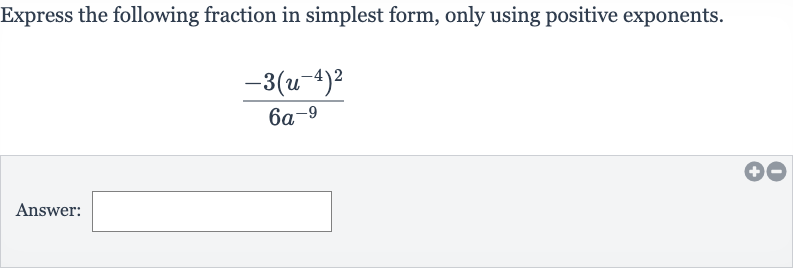
$Express the following fraction in simplest form, only using positive exponents. (-3(u^(-4))^(2))/(6a^(-9)) Answer:$

Express the following fraction in simplest form, only using positive exponents. $\newline$ $\frac{-3\left(u^{-4}\right)^{2}}{6 a^{-9}}$ $\newline$ Answer:

View step-by-step help

Home

Math Problems

Algebra 1

Multiplication with rational exponents

Full solution

Q. Express the following fraction in simplest form, only using positive exponents.

\newline

\frac{-3\left(u^{-4}\right)^{2}}{6 a^{-9}}

\newline

Answer:

Write Expression, Identify Exponents: Write down the given expression and identify the negative exponents. $\newline$ The given expression is $(-3(u^{-4})^{2})/(6a^{-9})$ . $\newline$ We need to simplify this expression and express it with only positive exponents.
Apply Power of Power Rule: Apply the power of a power rule to the term with the negative exponent in the numerator. $\newline$ The power of a power rule states that $(x^{m})^{n} = x^{m*n}$ . $\newline$ So, $(u^{-4})^{2} = u^{-4*2} = u^{-8}$ . $\newline$ Now the expression becomes $(-3u^{-8})/(6a^{-9})$ .
Convert Negative Exponents: Convert negative exponents to positive exponents by taking the reciprocal of the base. $\newline$ $u^{-8}$ becomes $\frac{1}{u^8}$ and $a^{-9}$ becomes $\frac{1}{a^9}$ . $\newline$ Now the expression becomes $\frac{-3}{u^8}/\frac{6}{a^9}$ .
Simplify Fraction: Simplify the fraction by multiplying the numerator and the denominator by the reciprocal of the denominator. $\newline$ This is equivalent to $(-3/u^{8}) \times (a^{9}/6)$ .
Multiply Numerators and Denominators: Multiply the numerators and the denominators. $(-3 \times a^9) / (u^8 \times 6)$ .
Divide by Greatest Common Divisor: Simplify the fraction by dividing both the numerator and the denominator by their greatest common divisor, which is $3$ in this case. $\left(-\frac{3}{3}\right) \cdot a^{9} / \left(u^{8} \cdot \left(\frac{6}{3}\right)\right).$ This simplifies to $\left(-1 \cdot a^{9}\right) / \left(u^{8} \cdot 2\right).$
Write Final Expression: Write the final simplified expression with only positive exponents. $\newline$ The final expression is $(-a^{9}) / (2u^{8})$ .