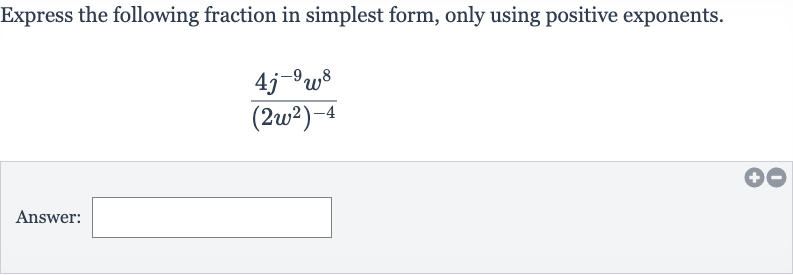
Express the following fraction in simplest form, only using positive exponents. (4j^(-9)w^(8))/((2w^(2))^(-4)) Answer:

Question

Express the following fraction in simplest form, only using positive exponents.  (4j^(-9)w^(8))/((2w^(2))^(-4)) Answer:

Bytelearn · Accepted Answer

Simplify Denominator: We start by simplifying the denominator. The denominator is (2w^(2))^(-4), which means we need to apply the negative exponent rule, which states that a^(-n) = 1/a^n. We will apply this rule to both 2 and w^(2).Apply Negative Exponent Rule: Applying the negative exponent rule to the denominator, we get: (2w^(2))^(-4) = 2^(-4) * (w^(2))^(-4) Now we will simplify each part separately.Simplify 2^(-4): Simplifying 2^(-4), we get: 2^(-4) = 1/2^4 = 1/16Simplify (w^(2))^(-4): Simplifying (w^(2))^(-4), we get: (w^(2))^(-4) = 1/(w^(2))^4 = 1/w^(8)Combine Denominator: Now we combine the simplified parts of the denominator: 2^(-4) * (w^(2))^(-4) = 1/16 * 1/w^(8) = 1/(16w^(8))Divide by Reciprocal: Next, we will simplify the entire fraction by dividing the numerator by the simplified denominator: (4j^(-9)w^(8)) / (1/(16w^(8)))Simplify Multiplication: When dividing by a fraction, it is equivalent to multiplying by its reciprocal. So we multiply the numerator by the reciprocal of the denominator: 4j^(-9)w^(8) * (16w^(8))Apply Exponent Rule: Now we simplify the multiplication: 4 * 16 * j^(-9) * w^(8) * w^(8)Combine Constants and Variables: Multiplying the constants 4 and 16, we get: 64 * j^(-9) * w^(8) * w^(8)Convert Negative Exponent: Next, we apply the exponent rule for multiplication, which states that a^(m) * a^(n) = a^(m+n) when multiplying like bases. We will apply this to w^(8) * w^(8): w^(8) * w^(8) = w^(8+8) = w^(16)Final Simplification: Now we combine the constants and the variables with their exponents: 64 * j^(-9) * w^(16)We want to express the fraction using only positive exponents. To do this, we apply the rule a^(-n) = 1/a^n to j^(-9): 64 * (1/j^9) * w^(16)Finally, we write the expression in simplest form: 64w^(16)/j^9

Express the following fraction in simplest form, only using positive exponents. $\newline$ $\frac{4 j^{-9} w^{8}}{\left(2 w^{2}\right)^{-4}}$ $\newline$ Answer:

Full solution

More problems from Multiplication with rational exponents

Related topics

Express the following fraction in simplest form, only using positive exponents.\newline4j−9w8(2w2)−4 \frac{4 j^{-9} w^{8}}{\left(2 w^{2}\right)^{-4}} (2w2)−44j−9w8​\newlineAnswer:

Express the following fraction in simplest form, only using positive exponents. $\newline$ $\frac{4 j^{-9} w^{8}}{\left(2 w^{2}\right)^{-4}}$ $\newline$ Answer: