Full solution
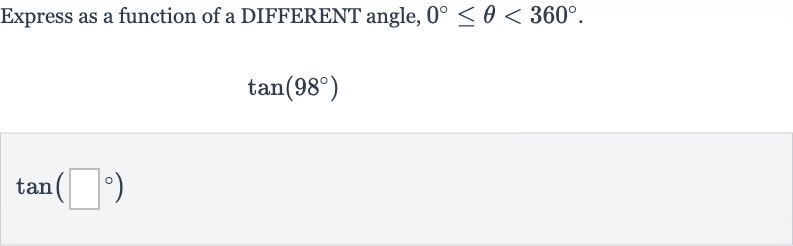
Q. Express as a function of a DIFFERENT angle, .
- Identify Reference Angle: Identify the reference angle for in the unit circle.Since is in the second quadrant where tangent is positive, we need to find the acute angle that is associated with . The reference angle is the difference between and , which is the straight line angle in the second quadrant.Reference angle = .
- Determine Tangent Sign: Determine the sign of the tangent function in the second quadrant.In the second quadrant, the sine function is positive and the cosine function is negative. Since tangent is sine over cosine, will be positive in the second quadrant.
- Express as Function: Express as a function of the reference angle.Since is positive in the second quadrant and we have found the reference angle to be , we can express as .
More problems from Find trigonometric ratios using reference angles
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
Question
. Find the value of in degrees.Write your answer in simplified, rationalized form. Do not round.____
Get tutor helpQuestionGet tutor help