Full solution
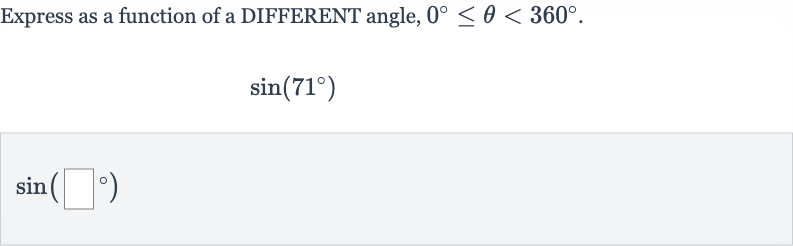
Q. Express as a function of a DIFFERENT angle, .
- Identify angle: Identify the related angle to that can be used to express as a function of a different angle.Since the sine function is periodic with a period of , we can find a related angle by subtracting from .Calculation:
- Calculate related angle: Express in terms of . Since for angles in the first and second quadrants, and for angles in the first and fourth quadrants, we can use the latter identity because is in the fourth quadrant. Therefore, . Calculation: , which confirms that .
More problems from Sin, cos, and tan of special angles
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
Question
. Find the value of in degrees.Write your answer in simplified, rationalized form. Do not round.____
Get tutor helpQuestionGet tutor help