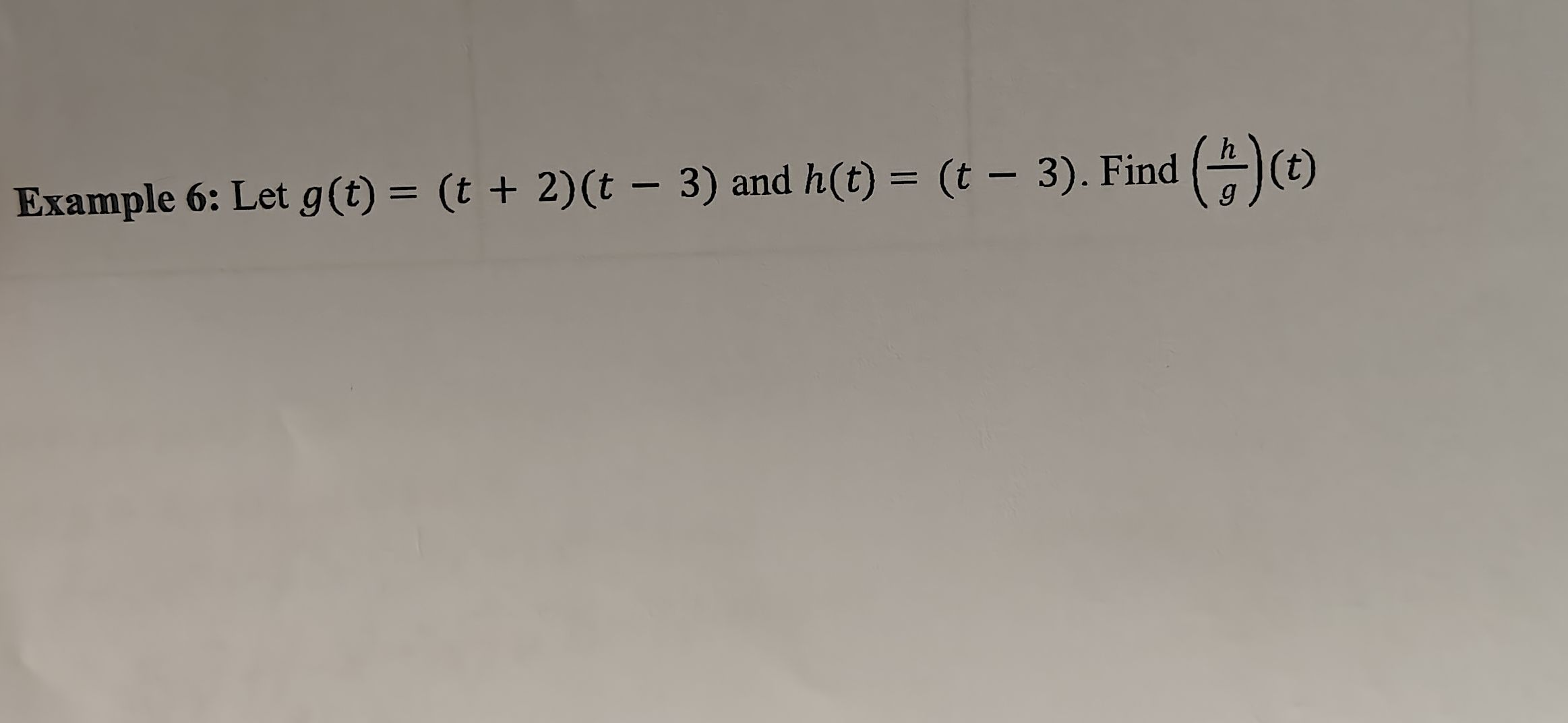AI tutor
Full solution
Q. Let and . Find
- Write Functions: First, let's write down the functions and that are given.
- Find : Now, we need to find the expression for , which means we will divide by .
- Substitute Expressions: Substitute the expressions for and into the equation.
- Simplify Expression: We can see that is a common factor in both the numerator and the denominator, so we can simplify the expression by canceling out .
- Final Answer: The simplified expression for is now , which is the final answer.