AI tutor
Full solution
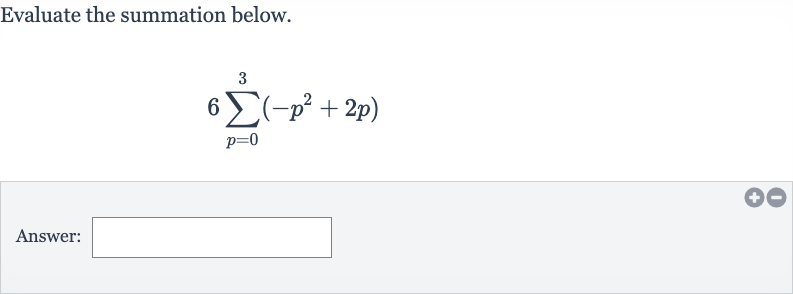
Q. Evaluate the summation below.Answer:
- Expand and Calculate: We start by expanding the summation expression for each value of from to .For : For : For : For :
- Sum up Results: Now, we sum up the results of the expression for each value of .Sum:
- Multiply by : Finally, we multiply the sum by as indicated by the summation expression .