AI tutor
Full solution
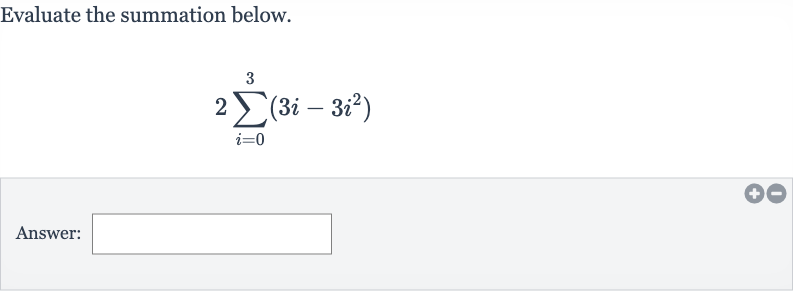
Q. Evaluate the summation below.Answer:
- Write Series Expression: Write down the series to be evaluated.We need to evaluate the summation of the series given by the expression . This means we will calculate the sum for each value of from to , multiply each term by , and then add up all the terms.
- Calculate Series Terms: Calculate the terms of the series for each value of .
For :
For :
For :
For : - Add Terms: Add up all the terms calculated in Step .Sum =
- Final Answer: State the final answer.The sum of the series is .