Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

Evaluate the left hand side to find the value of
a in the equation in simplest form.

(x^(4))/(x^((1)/(6)))=x^(a)
Answer:

Evaluate the left hand side to find the value of $a$ in the equation in simplest form. $\newline$ $\frac{x^{4}}{x^{\frac{1}{6}}}=x^{a}$ $\newline$ Answer:

View step-by-step help

View step-by-step help

Multiplication with rational exponents

Full solution

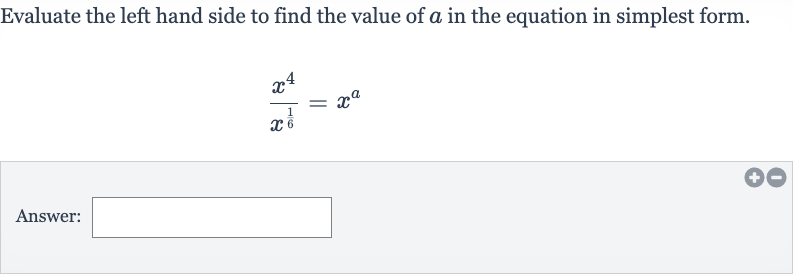
Q. Evaluate the left hand side to find the value of

a

in the equation in simplest form.

\newline

\frac{x^{4}}{x^{\frac{1}{6}}}=x^{a}

\newline

Answer:

Simplify using properties of exponents: Simplify the left-hand side of the equation using the properties of exponents. When dividing powers with the same base, subtract the exponents. $\frac{x^{4}}{x^{\frac{1}{6}}} = x^{4 - \frac{1}{6}}$
Subtract exponents: Perform the subtraction of the exponents. $\newline$ $4 - \left(\frac{1}{6}\right) = \left(\frac{24}{6}\right) - \left(\frac{1}{6}\right) = \frac{23}{6}$ $\newline$ So, $\left(x^{4}\right)/\left(x^{\left(\frac{1}{6}\right)}\right) = x^{\frac{23}{6}}$
Perform subtraction: Since the left-hand side of the equation is now simplified to $x^{\frac{23}{6}}$ , we can equate this to the right-hand side of the equation. $\newline$ $x^{\frac{23}{6}} = x^{a}$
Equating left and right sides: By the property of equality for exponential equations, if the bases are the same, then the exponents must be equal. $\newline$ Therefore, $a = \frac{23}{6}$

More problems from Multiplication with rational exponents

Question

\newline

81^{\frac{1}{2}}

\newline

Posted 9 months ago

Question

\newline

(-32)^{1/5}

\newline

Posted 9 months ago

Question

Simplify. Assume all variables are positive.

\newline

\frac{w^{\frac{4}{3}}}{w^{\frac{7}{3}}}

\newline

Write your answer in the form

A

\frac{A}{B}

A

B

are constants or variable expressions that have no variables in common. All exponents in your answer should be positive.

\newline

Posted 5 months ago

Question

Simplify. Assume all variables are positive.

\newline

(2r^{\frac{1}{3}})^8

\newline

\newline

Write your answer in the form

A

\frac{A}{B}

A

B

are constants or variable expressions that have no variables in common. All exponents in your answer should be positive.

\newline

Posted 5 months ago

Question

Simplify. Assume all variables are positive.

\newline

v^{\frac{7}{4}} \cdot v^{\frac{9}{4}}

\newline

\newline

Write your answer in the form

A

\frac{A}{B}

A

B

are constants or variable expressions that have no variables in common. All exponents in your answer should be positive.

\newline

\newline

Posted 5 months ago

Question

\newline

1^{\frac{1}{4}} \cdot 1^{\frac{1}{4}}

\newline

Posted 5 months ago

Question

\newline

32^{(-1/5)}

Posted 7 months ago

Question

Simplify. Assume all variables are positive.

\newline

\frac{w^{\frac{3}{2}}}{w^{\frac{5}{2}}}

\newline

\newline

Write your answer in the form

A

\frac{A}{B}

A

B

are constants or variable expressions that have no variables in common. All exponents in your answer should be positive.

\newline

\newline

Posted 9 months ago

Question

Simplify. Assume all variables are positive.

\newline

(27x)^{\frac{2}{3}}

\newline

\newline

Write your answer in the form

A

\frac{A}{B}

A

B

are constants or variable expressions that have no variables in common. All exponents in your answer should be positive.

\newline

Posted 8 months ago

Question

Simplify. Assume all variables are positive.

\newline

\frac{x^{\frac{1}{4}}}{x^{\frac{11}{4}}}

\newline

\newline

Write your answer in the form

A

\frac{A}{B}

A

B

are constants or variable expressions that have no variables in common. All exponents in your answer should be positive.

\newline

\newline

Posted 7 months ago

Related topics

Algebra - Order of Operations Algebra - Distributive Property `X` and `Y` Axes Geometry - Scalene Triangle Common Multiple Geometry - Quadrant