Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

Evaluate the left hand side to find the value of a in the equation in simplest form. (x^((2)/(3)))/(x^((1)/(2)))=x^(a) Answer:

Evaluate the left hand side to find the value of a a in the equation in simplest form.\newlinex23x12=xa \frac{x^{\frac{2}{3}}}{x^{\frac{1}{2}}}=x^{a} \newlineAnswer:

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Algebra 1right chevron icon
Write variable expressions for arithmetic sequencesright chevron icon

Full solution

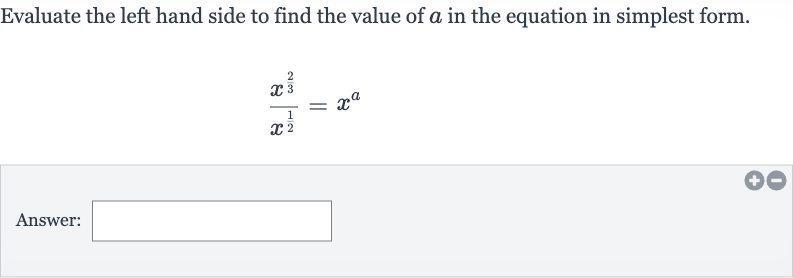
Q. Evaluate the left hand side to find the value of a a in the equation in simplest form.\newlinex23x12=xa \frac{x^{\frac{2}{3}}}{x^{\frac{1}{2}}}=x^{a} \newlineAnswer:
  1. Simplify Left-hand Side: To solve for aa, we need to simplify the left-hand side of the equation using the properties of exponents.\newlineWhen dividing powers with the same base, we subtract the exponents: xm/xn=x(m−n)x^m / x^n = x^{(m-n)}.\newlineLet's apply this rule to the given expression.
  2. Subtract Exponents: We have x23x^{\frac{2}{3}} divided by x12x^{\frac{1}{2}}.\newlineSubtract the exponents: (23)−(12)\left(\frac{2}{3}\right) - \left(\frac{1}{2}\right).\newlineTo subtract these fractions, we need a common denominator.\newlineThe common denominator for 33 and 22 is 66.
  3. Find Common Denominator: Convert (23)(\frac{2}{3}) and (12)(\frac{1}{2}) to have the common denominator of 66.\newline(23)(\frac{2}{3}) becomes 46\frac{4}{6} and (12)(\frac{1}{2}) becomes 36\frac{3}{6}.\newlineNow subtract the fractions: 46−36=16\frac{4}{6} - \frac{3}{6} = \frac{1}{6}.
  4. Convert Fractions: So, the simplified form of the left-hand side is x16x^{\frac{1}{6}}.\newlineTherefore, a=16a = \frac{1}{6}.\newlineThis is the simplest form of the exponent for the given expression.

More problems from Write variable expressions for arithmetic sequences

Question
Find the sum of the finite arithmetic series. ∑n=110(7n+4)\sum_{n=1}^{10} (7n+4)\newline______
Get tutor helpright-arrow

Posted 9 months ago

Question
Type the missing number in this sequence: `55,\59,\63,\text{_____},\71,\75,\79`
Get tutor helpright-arrow

Posted 5 months ago

Question
Type the missing number in this sequence: `2, 4 8`, ______,`32`
Get tutor helpright-arrow

Posted 5 months ago

Question
What kind of sequence is this? 2,10,50,250,…2, 10, 50, 250, \ldots Choices:Choices:\newline[A]arithmetic\text{[A]arithmetic}\newline[B]geometric\text{[B]geometric}\newline[C]both\text{[C]both}\newline[D]neither\text{[D]neither}
Get tutor helpright-arrow

Posted 5 months ago

Question
What is the missing number in this pattern? 1,4,9,16,25,36,49,64,81,____1, 4, 9, 16, 25, 36, 49, 64, 81, \_\_\_\_
Get tutor helpright-arrow

Posted 7 months ago

Question
Classify the series. ∑n=012(n+2)3 \sum_{n = 0}^{12} (n + 2)^3 \newlineChoices:\newline[A]arithmetic\text{[A]arithmetic}\newline[B]geometric\text{[B]geometric}\newline[C]both\text{[C]both}\newline[D]neither\text{[D]neither}
Get tutor helpright-arrow

Posted 5 months ago

Question
Find the first three partial sums of the series.\newline1+6+11+16+21+26+⋯1 + 6 + 11 + 16 + 21 + 26 + \cdots\newlineWrite your answers as integers or fractions in simplest form.\newlineS1=S_1 = ____\newlineS2=S_2 = ____\newlineS3=S_3 = ____
Get tutor helpright-arrow

Posted 7 months ago

Question
Find the third partial sum of the series. \newline3+9+15+21+27+33+⋯3 + 9 + 15 + 21 + 27 + 33 + \cdots\newlineWrite your answer as an integer or a fraction in simplest form. \newlineS3=S_3 = ____
Get tutor helpright-arrow

Posted 7 months ago

Question
Find the first three partial sums of the series. \newline1+7+13+19+25+31+⋯1 + 7 + 13 + 19 + 25 + 31 + \cdots\newlineWrite your answers as integers or fractions in simplest form. \newlineS1=S_1 = ____\newlineS2=S_2 = ____\newlineS3=S_3 = ____
Get tutor helpright-arrow

Posted 9 months ago

Question
Does the infinite geometric series converge or diverge?\newline1+34+916+2764+⋯1 + \frac{3}{4} + \frac{9}{16} + \frac{27}{64} + \cdots\newlineChoices:\newline[A]converge\text{[A]converge}\newline[B]diverge\text{[B]diverge}
Get tutor helpright-arrow

Posted 5 months ago

View all (75)

Related topics

Algebra - Order of OperationsAlgebra - Distributive Property`X` and `Y` AxesGeometry - Scalene TriangleCommon MultipleGeometry - Quadrant