Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

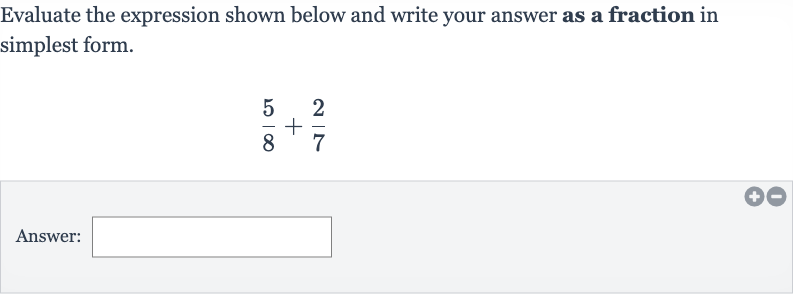
Evaluate the expression shown below and write your answer as a fraction in simplest form. (5)/(8)+(2)/(7) Answer:

Evaluate the expression shown below and write your answer as a fraction in simplest form.\newline58+27 \frac{5}{8}+\frac{2}{7} \newlineAnswer:

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Algebra 2right chevron icon
Roots of rational numbersright chevron icon

Full solution

Q. Evaluate the expression shown below and write your answer as a fraction in simplest form.\newline58+27 \frac{5}{8}+\frac{2}{7} \newlineAnswer:
  1. Identify LCD: Identify the Least Common Denominator (LCD) for the fractions 58\frac{5}{8} and 27\frac{2}{7}. The denominators are 88 and 77, which are both prime numbers. Therefore, the LCD is their product, which is 8×7=568 \times 7 = 56.
  2. Convert to Equivalent Fraction: Convert each fraction to an equivalent fraction with the LCD as the denominator.\newlineFor 58\frac{5}{8}, we multiply the numerator and denominator by 77 to get (5×7)/(8×7)=3556\left(5 \times 7\right) / \left(8 \times 7\right) = \frac{35}{56}.\newlineFor 27\frac{2}{7}, we multiply the numerator and denominator by 88 to get (2×8)/(7×8)=1656\left(2 \times 8\right) / \left(7 \times 8\right) = \frac{16}{56}.
  3. Add Fractions: Add the equivalent fractions.\newlineNow that both fractions have the same denominator, we can add them directly:\newline3556+1656=(35+16)56=5156\frac{35}{56} + \frac{16}{56} = \frac{(35 + 16)}{56} = \frac{51}{56}.

More problems from Roots of rational numbers

Question
Find the real-number root. \newline16\sqrt{16}\newline\newlineWrite your answer in simplified form. \newline____\_\_\_\_
Get tutor helpright-arrow

Posted 9 months ago

Question
Find the real-number root. \newline916\sqrt{\frac{9}{16}}\newlineSimplify your answer and write it as a decimal or as a proper or improper fraction.\newline_____\_\_\_\_\_
Get tutor helpright-arrow

Posted 9 months ago

Question
Find the real-number root using a calculator. Round your answer to the nearest thousandth. \newline`\sqrt{957} `\newline _______
Get tutor helpright-arrow

Posted 9 months ago

Question
Multiply. Write your answer in simplest form. \newline273×42\sqrt{273} \times \sqrt{42}\newline______
Get tutor helpright-arrow

Posted 7 months ago

Question
Simplify. \newline634\sqrt{\frac{63}{4}}\newline______
Get tutor helpright-arrow

Posted 9 months ago

Question
Simplify. Rationalize the denominator.\newline−49−2\frac{-4}{9 - \sqrt{2}}\newline______
Get tutor helpright-arrow

Posted 9 months ago

Question
Solve for z z .\newline16=z 16 = \sqrt{z} \newlinez= z = _____
Get tutor helpright-arrow

Posted 9 months ago

Question
Simplify the radical expression. 14x14 \sqrt{14x^{14}} Write your answer in the form A A , B \sqrt{B} , or AB A\sqrt{B} , where A A and B B are constants or expressions in x x . Use at most one radical in your answer, and at most one absolute value symbol in your expression for A A . ______
Get tutor helpright-arrow

Posted 9 months ago

Question
Simplify the radical expression.\newline12x12\sqrt{12x^{12}}\newlineWrite your answer in the form AA, B\sqrt{B}, or ABA\sqrt{B}, where AA and BB are constants or expressions in xx. Use at most one radical in your answer, and at most one absolute value symbol in your expression for AA.\newline‾\underline{\hspace{3cm}}
Get tutor helpright-arrow

Posted 9 months ago

Question
What is the value of sin⁡(5π6)? \sin \left(\frac{5 \pi}{6}\right) ? \newlineChoose 11 answer:\newline(A) −32 -\frac{\sqrt{3}}{2} \newline(B) −22 -\frac{\sqrt{2}}{2} \newline(C) 12 \frac{1}{2} \newline(D) 150150
Get tutor helpright-arrow

Posted 10 months ago

View all (169)

Related topics

Algebra - Order of OperationsAlgebra - Distributive Property`X` and `Y` AxesGeometry - Scalene TriangleCommon MultipleGeometry - Quadrant