Resources

Resources

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

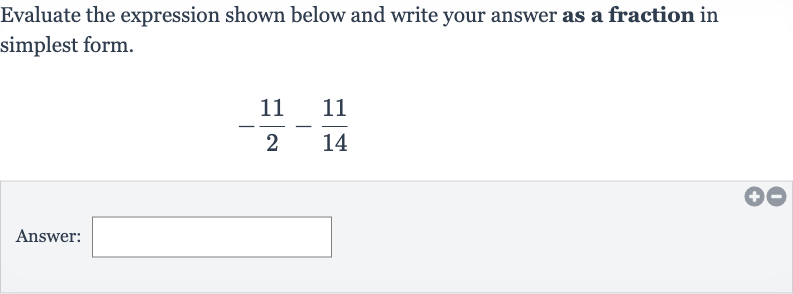
$Evaluate the expression shown below and write your answer as a fraction in simplest form. -(11)/(2)-(11)/(14) Answer:$

Evaluate the expression shown below and write your answer as a fraction in simplest form. $\newline$ $-\frac{11}{2}-\frac{11}{14}$ $\newline$ Answer:

Full solution

Q. Evaluate the expression shown below and write your answer as a fraction in simplest form.

\newline

-\frac{11}{2}-\frac{11}{14}

\newline

Answer:

Identify LCD: Identify the least common denominator (LCD) for the fractions $-\frac{11}{2}$ and $-\frac{11}{14}$ . The denominators are $2$ and $14$ . The LCD of $2$ and $14$ is $14$ because $14$ is the smallest number that both $2$ and $14$ can divide into without leaving a remainder.
Convert to Equivalent Fraction: Convert the fraction with the denominator of $2$ to an equivalent fraction with the denominator of $14$ . To do this, multiply both the numerator and the denominator of $-\frac{11}{2}$ by $7$ (since $14$ divided by $2$ is $7$ ). $-\frac{11}{2} \times \frac{7}{7} = -\frac{77}{14}$
Combine Fractions: Now that both fractions have the same denominator, you can combine them by adding their numerators. $\newline$ $-\frac{77}{14} - \frac{11}{14} = \frac{(-77 - 11)}{14}$
Perform Subtraction: Perform the subtraction in the numerator. $\newline$ $-77 - 11 = -88$ $\newline$ So, the combined fraction is $-\frac{88}{14}$ .
Simplify Fraction: Simplify the fraction $-\frac{88}{14}$ by dividing both the numerator and the denominator by their greatest common divisor (GCD). $\newline$ The GCD of $88$ and $14$ is $2$ . $\newline$ $-\frac{88}{14} \div \frac{2}{2} = -\frac{44}{7}$