AI tutor
Full solution
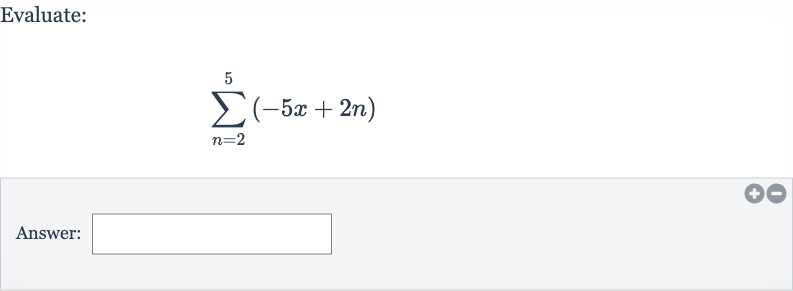
Q. Evaluate:Answer:
- Evaluate Expression for : We need to evaluate the sum of the series from to for the expression . We will do this by calculating the value of the expression for each value of and then summing those values.
- Evaluate Expression for : First, let's find the value of the expression when : .
- Evaluate Expression for : Next, find the value of the expression when : .
- Evaluate Expression for : Now, find the value of the expression when : .
- Sum Values of Expression: Finally, find the value of the expression when : .
- Combine Like Terms: Now we sum the values of the expression for each : .
- Final Result: Combine like terms: .
- Final Result: Combine like terms: .The sum of the series from to of the expression is .