AI tutor
Full solution
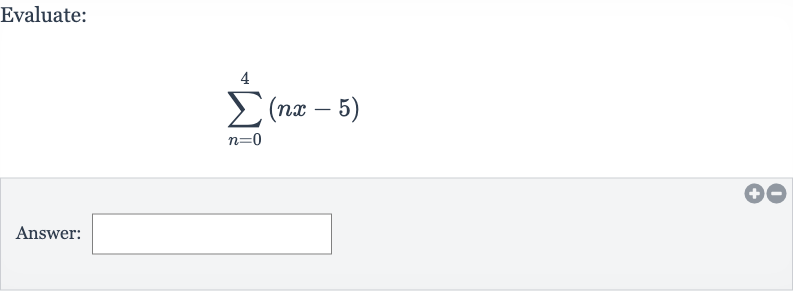
Q. Evaluate:Answer:
- Understand the expression: Understand the expressionThe expression means we need to calculate the sum of the terms for each integer value of from to .
- Evaluate : Evaluate the expression for When , the term is which simplifies to .
- Evaluate : Evaluate the expression for When , the term is which simplifies to .
- Evaluate : Evaluate the expression for When , the term is which simplifies to .
- Evaluate : Evaluate the expression for When , the term is which simplifies to .
- Evaluate : Evaluate the expression for When , the term is which simplifies to .
- Add all terms: Add all the terms togetherNow we add all the terms from to :
- Combine like terms: Combine like termsCombine the terms and the constant terms:This simplifies to .
- Verify final answer: Verify the final answerThe final expression is the sum of the terms from to .
More problems from Evaluate rational expressions II
QuestionGet tutor help