AI tutor
Welcome to Bytelearn!
Let’s check out your problem:
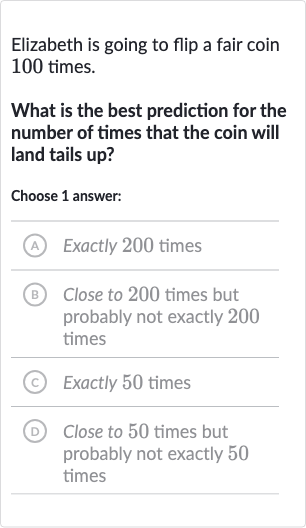
Elizabeth is going to flip a fair coin times.What is the best prediction for the number of times that the coin will land tails up?Choose answer:(A) Exactly times(B) Close to times but probably not exactly times(C) Exactly times(D) Close to times but probably not exactly times
Full solution
Q. Elizabeth is going to flip a fair coin times.What is the best prediction for the number of times that the coin will land tails up?Choose answer:(A) Exactly times(B) Close to times but probably not exactly times(C) Exactly times(D) Close to times but probably not exactly times
- Understand single event probability: We need to understand the probability of a single event first. A fair coin has two sides, heads and tails, so the probability of getting tails on a single flip is .
- Expectation over large number: Since the coin is fair, we expect heads and tails to occur with equal frequency over a large number of flips. Therefore, we can predict that half of the flips will result in tails.
- Calculate expected number of tails: To find the expected number of tails, we multiply the total number of flips by the probability of getting tails on a single flip. This is flips probability of tails expected tails.
- Choose best answer from options: Now we need to choose the best answer from the options given. Option (A) "Exactly times" is not possible because we are only flipping the coin times. Option (B) "Close to times but probably not exactly times" is also incorrect for the same reason. Option (C) "Exactly times" is a possibility, but due to the nature of probability, it is unlikely to be exactly times. Option (D) "Close to times but probably not exactly times" is the most accurate prediction, as it accounts for the variability inherent in random events.
- Best prediction for number of tails: Therefore, the best prediction for the number of times the coin will land tails up is close to times but probably not exactly times, which corresponds to option .


