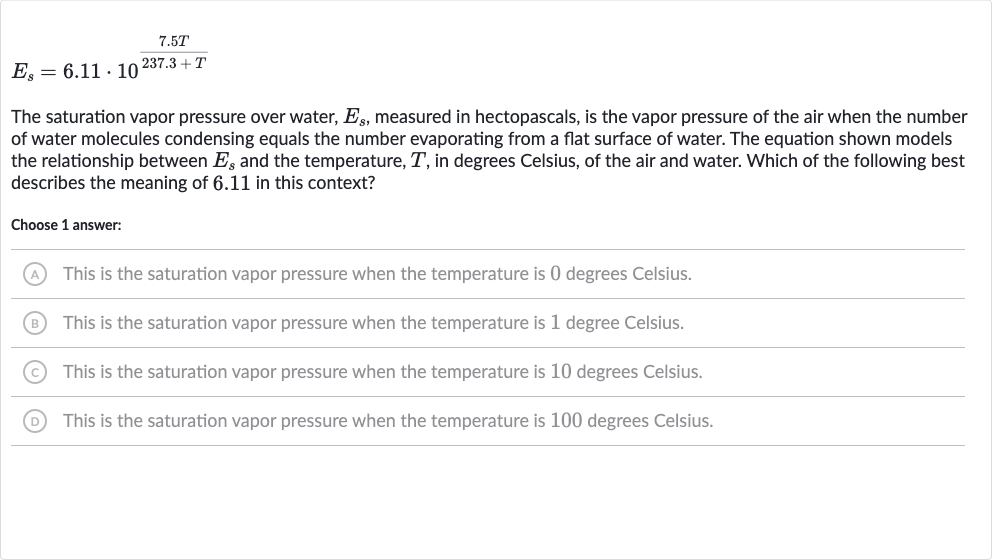
E_(s)=6.11*10^((7.5 T)/(237.3+T)) The saturation vapor pressure over water, E_(s), measured in hectopascals, is the vapor pressure of the air when the number of water molecules condensing equals the number evaporating from a flat surface of water. The equation shown models the relationship between E_(s) and the temperature, T, in degrees Celsius, of the air and water. Which of the following best describes the meaning of 6.11 in this context? Choose 1 answer: (A) This is the saturation vapor pressure when the temperature is 0 degrees Celsius. (B) This is the saturation vapor pressure when the temperature is 1 degree Celsius. (C) This is the saturation vapor pressure when the temperature is 10 degrees Celsius. (D) This is the saturation vapor pressure when the temperature is 100 degrees Celsius.

Question

E_(s)=6.11*10^((7.5 T)/(237.3+T)) The saturation vapor pressure over water,  E_(s), measured in hectopascals, is the vapor pressure of the air when the number of water molecules condensing equals the number evaporating from a flat surface of water. The equation shown models the relationship between  E_(s) and the temperature,  T, in degrees Celsius, of the air and water. Which of the following best describes the meaning of 6.11 in this context? Choose 1 answer: (A) This is the saturation vapor pressure when the temperature is 0 degrees Celsius. (B) This is the saturation vapor pressure when the temperature is 1 degree Celsius. (C) This is the saturation vapor pressure when the temperature is 10 degrees Celsius. (D) This is the saturation vapor pressure when the temperature is 100 degrees Celsius.

Bytelearn · Accepted Answer

Understand Equation and Constant: Understand the equation and identify the constant. The equation E_(s)=6.11*10^((7.5*T)/(237.3+T)) models the relationship between the saturation vapor pressure E_(s) and the temperature T in degrees Celsius. The constant 6.11 is a part of this equation, and we need to determine its significance.Analyze Role of 6.11: Analyze the equation to determine the role of 6.11. To understand the meaning of 6.11, we need to consider the equation at a specific temperature. The most logical temperature to consider is 0 degrees Celsius, as it is a standard reference point for many scientific measurements.Substitute T=0: Substitute T=0 into the equation to see the effect on E_(s). If we substitute T=0 into the equation, we get E_(s)=6.11*10^((7.5*0)/(237.3+0)). This simplifies to E_(s)=6.11*10^(0), since any number to the power of 0 is 1.Calculate Vapor Pressure: Calculate the saturation vapor pressure at 0 degrees Celsius. Now, we calculate E_(s) with T=0: E_(s)=6.11*10^(0) = 6.11*1 = 6.11. This means that the saturation vapor pressure at 0 degrees Celsius is 6.11 hectopascals.

Full solution

More problems from Interpreting Linear Expressions

Related topics