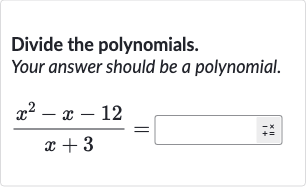
Divide the polynomials. Your answer should be a polynomial. (x^(2)-x-12)/(x+3)=◻

Question

Divide the polynomials. Your answer should be a polynomial.  (x^(2)-x-12)/(x+3)=◻

Bytelearn · Accepted Answer

Set up division format: Set up the division of the polynomials in long division format. We want to divide x^2 - x - 12 by x + 3.Divide first terms: Divide the first term of the dividend, x^2, by the first term of the divisor, x, to get the first term of the quotient. x^2 divided by x is x. Write x above the division bar.Multiply and subtract: Multiply the divisor, x + 3, by the first term of the quotient, x, to get x(x + 3) = x^2 + 3x. Subtract this from the dividend under the division bar.Perform subtraction: Perform the subtraction step by subtracting (x^2 + 3x) from (x^2 - x). x^2 - x - (x^2 + 3x) = -x - 3x = -4x. Bring down the next term of the dividend, which is -12, to get -4x - 12.Bring down next term: Divide the new first term of the remaining dividend, -4x, by the first term of the divisor, x, to get the next term of the quotient. -4x divided by x is -4. Write -4 next to x above the division bar, making the quotient x - 4.Divide new first term: Multiply the divisor, x + 3, by the new term of the quotient, -4, to get -4(x + 3) = -4x - 12. Subtract this from the remaining dividend under the division bar.Multiply and subtract: Perform the subtraction step by subtracting (-4x - 12) from (-4x - 12). -4x - 12 - (-4x - 12) = 0. There is no remainder, so the division is complete.

Divide the polynomials. $\newline$ Your answer should be a polynomial. $\newline$ $\frac{x^{2}-x-12}{x+3}=$

Full solution

More problems from Factor sums and differences of cubes

Related topics

Divide the polynomials.\newlineYour answer should be a polynomial.\newlinex2−x−12x+3= \frac{x^{2}-x-12}{x+3}= x+3x2−x−12​=

Divide the polynomials. $\newline$ Your answer should be a polynomial. $\newline$ $\frac{x^{2}-x-12}{x+3}=$