Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

Determine the value of y, if x is 25 . y=sqrt(x+11) Answer: y=

Determine the value of y y , if x x is 2525 .\newliney=x+11 y=\sqrt{x+11} \newlineAnswer: y= y=

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Precalculusright chevron icon
Find trigonometric ratios using multiple identitiesright chevron icon

Full solution

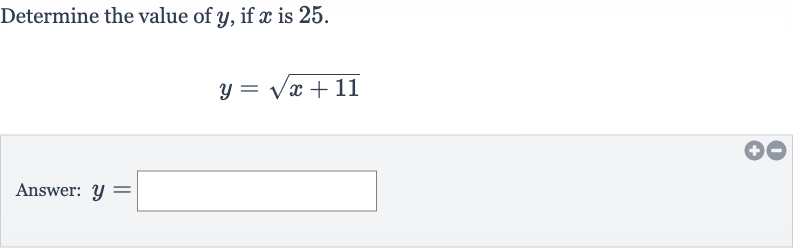
Q. Determine the value of y y , if x x is 2525 .\newliney=x+11 y=\sqrt{x+11} \newlineAnswer: y= y=
  1. Identify Values and Equation: Identify the given values and the equation to solve for yy. We are given that x=25x = 25 and the equation y=x+11y = \sqrt{x + 11}. We need to substitute the value of xx into the equation to find yy.
  2. Substitute Value into Equation: Substitute the value of xx into the equation.\newlineSubstitute x=25x = 25 into y=x+11y = \sqrt{x + 11} to get y=25+11y = \sqrt{25 + 11}.
  3. Perform Addition Inside Square Root: Perform the addition inside the square root.\newlineCalculate the sum inside the square root: 25+11=3625 + 11 = 36.
  4. Calculate Square Root: Calculate the square root of the sum to find the value of yy. Find the square root of 3636, which is y=36y = \sqrt{36}.
  5. Determine Final Value: Determine the square root of 3636. Since the square root of 3636 is 66, we have y=6y = 6.

More problems from Find trigonometric ratios using multiple identities

Question
If cos⁡(θ)=817 \cos(\theta)=\frac{8}{17} and 0∘<θ<90∘ 0^\circ<\theta<90^\circ , what is sec⁡(θ) \sec(\theta) ? Write your answer in simplified, rationalized form. sec⁡(θ)= \sec(\theta)= ______
Get tutor helpright-arrow

Posted 3 months ago

Question
cos⁡(x)=−0.3\cos(x) = -0.3. What is sin⁡(90∘−x)\sin(90^\circ - x)? ____
Get tutor helpright-arrow

Posted 3 months ago

Question
If csc⁡(θ)=178 \csc(\theta) = \frac{17}{8} and 0∘<θ<90∘ 0^\circ < \theta < 90^\circ , what is tan⁡(θ) \tan(\theta) ?\newlineWrite your answer in simplified, rationalized form.\newlinetan⁡(θ)= \tan(\theta) = ______\newline
Get tutor helpright-arrow

Posted 3 months ago

Question
Is the sine function even or odd?\newlineChoices:\newline[A]even\text{[A]even}\newline[B]odd\text{[B]odd}
Get tutor helpright-arrow

Posted 3 months ago

Question
Write the sentence as an equation. \newline8989 is equal to the quotient of yy and 1717 \newlineType a slash ( / / ) if you want to use a division sign. \newline_____
Get tutor helpright-arrow

Posted 2 months ago

Question
Write the sentence as an equation. \newline4545 is equal to the quotient of zz and 55 \newlineType a slash ( / / ) if you want to use a division sign. \newline_____
Get tutor helpright-arrow

Posted 4 months ago

Question
Solve for hh. \newline h2+24h=0h^2 + 24h = 0 \newlineWrite each solution as an integer, proper fraction, or improper fraction in simplest form. If there are multiple solutions, separate them with commas. \newline h=h = _____
Get tutor helpright-arrow

Posted 4 months ago

Question
h2−4h=0h^2 - 4h = 0
Get tutor helpright-arrow

Posted 2 months ago

Question
Combine the like terms to create an equivalent expression:\newline−k−(−8k)=□-k-(-8k)=\square
Get tutor helpright-arrow

Posted 4 months ago

Question
Which of the following degree measures is equal to 5π 5 \pi radians?\newline(The number of degrees of arc in a circle is 360360 . The number of radians of arc in a circle is 2π 2 \pi .)\newlineChoose 11 answer:\newline(A) 144∘ 144^{\circ} \newline(B) 900∘ 900^{\circ} \newline(C) 1,080∘ 1,080^{\circ} \newline(D) 1,800∘ 1,800^{\circ}
Get tutor helpright-arrow

Posted 2 months ago

View all (70)