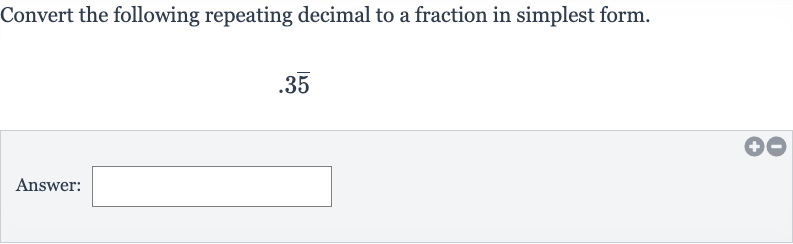
Convert the following repeating decimal to a fraction in simplest form. .3 bar(5) Answer:

Question

Convert the following repeating decimal to a fraction in simplest form.  .3 bar(5) Answer:

Bytelearn · Accepted Answer

Define x as repeating decimal: Let x be the repeating decimal 0.3 with a repeating 5, so x = 0.35555... We need to find an equation that we can solve for x that will eliminate the repeating decimal.Multiply by 10: Multiply x by 10 to shift the decimal point one place to the right, which gives us 10x = 3.5555... This will help us set up an equation to eliminate the repeating part.Multiply by 100: Multiply x by 100 to shift the decimal point two places to the right, which gives us 100x = 35.5555... Now we have two equations: 10x = 3.5555... and 100x = 35.5555...Subtract equations: Subtract the equation 10x = 3.5555... from the equation 100x = 35.5555... to get 90x = 32. This subtraction eliminates the repeating decimal and gives us an equation we can solve for x.Divide by 90: Divide both sides of the equation 90x = 32 by 90 to solve for x, which gives us x = 32/90. Now we have the decimal as a fraction, but it may not be in simplest form.Simplify fraction: Simplify the fraction 32/90 by finding the greatest common divisor (GCD) of 32 and 90. The GCD of 32 and 90 is 2.Find GCD and divide: Divide both the numerator and the denominator by the GCD (2) to simplify the fraction, which gives us x = (32/2)/(90/2) = 16/45. Now we have the fraction in simplest form.

Convert the following repeating decimal to a fraction in simplest form. $\newline$ $.3 \overline{5}$ $\newline$ Answer:

Full solution

More problems from Partial sums of geometric series

Related topics

Convert the following repeating decimal to a fraction in simplest form.\newline.35‾ .3 \overline{5} .35\newlineAnswer:

Convert the following repeating decimal to a fraction in simplest form. $\newline$ $.3 \overline{5}$ $\newline$ Answer: