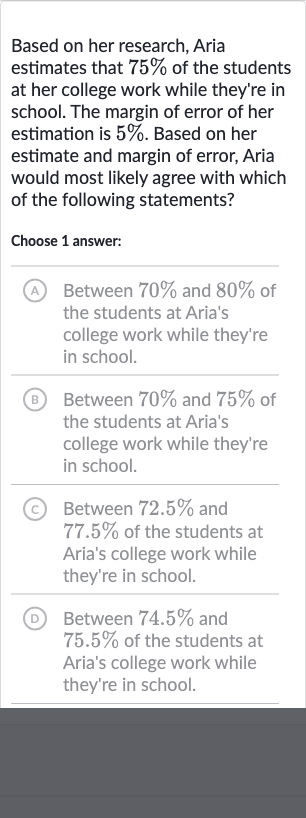
Based on her research, Aria estimates that 75% of the students at her college work while they're in school. The margin of error of her estimation is 5%. Based on her estimate and margin of error, Aria would most likely agree with which of the following statements? Choose 1 answer: (A) Between 70% and 80% of the students at Aria's college work while they're in school. (B) Between 70% and 75% of the students at Aria's college work while they're in school. (C) Between 72.5% and 77.5% of the students at Aria's college work while they're in school. (D) Between 74.5% and 75.5% of the students at Aria's college work while they're in school.

Question

Bytelearn · Accepted Answer

Estimate Range Calculation: Aria's estimate is that 75% of the students work while they're in school, and the margin of error is 5%. To find the range of percentages, we need to add and subtract the margin of error from the estimate.Calculate Lower Limit: Calculate the lower limit of the range by subtracting the margin of error from the estimate. Lower limit = Estimate - Margin of error Lower limit = 75% - 5% Lower limit = 70%Calculate Upper Limit: Calculate the upper limit of the range by adding the margin of error to the estimate. Upper limit = Estimate + Margin of error Upper limit = 75% + 5% Upper limit = 80%Final Conclusion: Based on the calculations, Aria would agree that between 70% and 80% of the students at her college work while they're in school.

Full solution

More problems from Interpret confidence intervals for population means

Related topics