AI tutor
Full solution
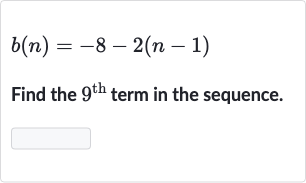
Q. Find the term in the sequence.
- Understanding the sequence formula: Understand the sequence formula.The given sequence formula is . This is an arithmetic sequence where the first term is when .
- Plugging in the value for the th term: Plug in the value for the term.To find the term, we substitute into the sequence formula: .
- Simplifying the expression: Simplify the expression.Simplify the expression inside the parentheses first: .
- Performing the multiplication: Perform the multiplication.Now multiply by : .
- Combining like terms: Combine like terms.Add the numbers together to get the term: .