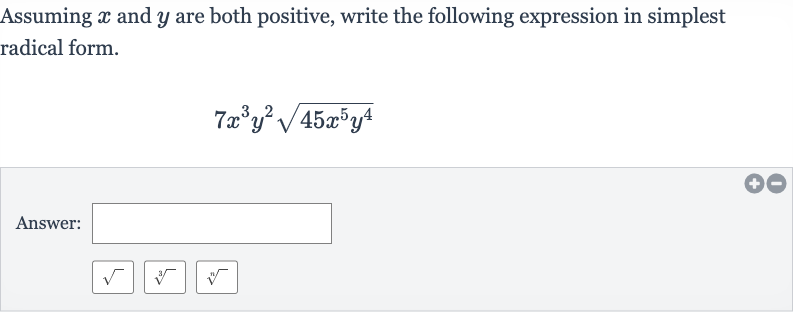
Assuming x and y are both positive, write the following expression in simplest radical form. 7x^(3)y^(2)sqrt(45x^(5)y^(4)) Answer:

Question

Assuming  x and  y are both positive, write the following expression in simplest radical form.  7x^(3)y^(2)sqrt(45x^(5)y^(4)) Answer:

Bytelearn · Accepted Answer

Identify Parts for Simplification: Identify the parts of the expression that can be simplified. We have the expression 7x^(3)y^(2)sqrt(45x^(5)y^(4)). The square root can be simplified by taking out factors that are perfect squares.Simplify Square Root: Simplify the square root. sqrt(45x^(5)y^(4)) can be broken down into sqrt(9 * 5 * x^(4) * x * y^(4)). Since 9, x^(4), and y^(4) are perfect squares, we can take the square root of these separately. sqrt(9) = 3, sqrt(x^(4)) = x^(2), and sqrt(y^(4)) = y^(2). So, sqrt(45x^(5)y^(4)) = 3x^(2)y^(2)sqrt(5x).Combine Simplified Terms: Combine the simplified square root with the rest of the expression. Now we have 7x^(3)y^(2) * 3x^(2)y^(2)sqrt(5x). Multiplying the coefficients (7 and 3) and the like terms (x^(3) and x^(2), y^(2) and y^(2)) gives us: 7 * 3 * x^(3+2) * y^(2+2) * sqrt(5x) = 21x^(5)y^(4)sqrt(5x).Check for Further Simplifications: Check for any further simplifications. The expression 21x^(5)y^(4)sqrt(5x) is already in its simplest radical form. There are no further simplifications that can be made since 5x is not a perfect square and cannot be taken out of the square root.

Assuming $x$ and $y$ are both positive, write the following expression in simplest radical form. $\newline$ $7 x^{3} y^{2} \sqrt{45 x^{5} y^{4}}$ $\newline$ Answer:

Full solution

More problems from Simplify radical expressions with root inside the root

Related topics

Assuming x x x and y y y are both positive, write the following expression in simplest radical form.\newline7x3y245x5y4 7 x^{3} y^{2} \sqrt{45 x^{5} y^{4}} 7x3y245x5y4​\newlineAnswer:

Assuming $x$ and $y$ are both positive, write the following expression in simplest radical form. $\newline$ $7 x^{3} y^{2} \sqrt{45 x^{5} y^{4}}$ $\newline$ Answer: