Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

Assuming
x and
y are both positive, write the following expression in simplest radical form.

3sqrt(28x^(2)y^(3))
Answer:

Assuming $x$ and $y$ are both positive, write the following expression in simplest radical form. $\newline$ $3 \sqrt{28 x^{2} y^{3}}$ $\newline$ Answer:

View step-by-step help

View step-by-step help

Simplify the product of two radical expressions having same variable

Full solution

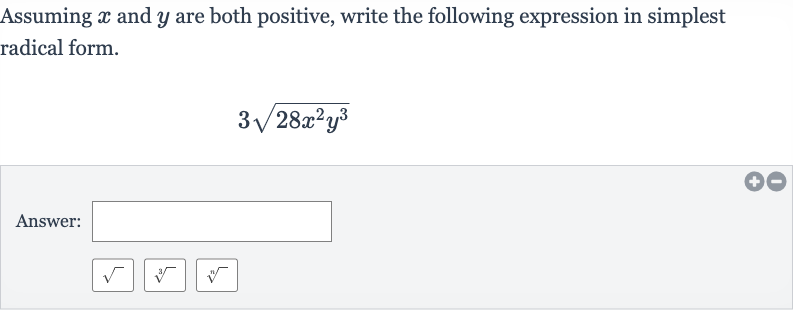
Q. Assuming

x

and

y

are both positive, write the following expression in simplest radical form.

\newline

3 \sqrt{28 x^{2} y^{3}}

\newline

Answer:

Simplify Radicand: Simplify the radicand (the number inside the radical). We have $28x^2y^3$ under the square root. The number $28$ can be factored into $4$ and $7$ , where $4$ is a perfect square. Also, $x^2$ is a perfect square, and $y^3$ can be split into $y^2$ (a perfect square) and $y$ . $3\sqrt{28x^2y^3} = 3\sqrt{4\cdot7\cdot x^2\cdot y^2\cdot y}$
Take Out Perfect Squares: Take out the perfect squares from under the radical. $\newline$ Since $4$ , $x^2$ , and $y^2$ are perfect squares, we can take their square roots out of the radical. $\newline$ $3\sqrt{4\cdot7\cdot x^2\cdot y^2\cdot y} = 3\cdot2\cdot x\cdot y\cdot\sqrt{7y}$ $\newline$ $3\sqrt{28x^2y^3} = 6xy\cdot\sqrt{7y}$
Combine Constants and Variables: Combine the constants and variables outside the radical. We multiply the constants and variables that are outside the radical to simplify the expression. $6xy\sqrt{7y}$ This is already simplified, and there are no further simplifications needed.

More problems from Simplify the product of two radical expressions having same variable

Question

Find the real-number root.

\newline

\sqrt{16}

\newline

\newline

Write your answer in simplified form.

\newline

\_\_\_\_

Posted 2 months ago

Question

Find the real-number root.

\newline

\sqrt{\frac{9}{16}}

\newline

Simplify your answer and write it as a decimal or as a proper or improper fraction.

\newline

\_\_\_\_\_

Posted 2 months ago

Question

Find the real-number root using a calculator. Round your answer to the nearest thousandth.

\newline

\newline

Posted 2 months ago

Question

Multiply. Write your answer in simplest form.

\newline

\sqrt{273} \times \sqrt{42}

\newline

Posted 1 month ago

Question

\newline

\sqrt{\frac{63}{4}}

\newline

Posted 2 months ago

Question

Simplify. Rationalize the denominator.

\newline

\frac{-4}{9 - \sqrt{2}}

\newline

Posted 2 months ago

Question

z

\newline

16 = \sqrt{z}

\newline

z =

Posted 2 months ago

Question

Simplify the radical expression.

\sqrt{14x^{14}}

Write your answer in the form

A

\sqrt{B}

A\sqrt{B}

A

B

are constants or expressions in

x

. Use at most one radical in your answer, and at most one absolute value symbol in your expression for

A

Posted 2 months ago

Question

Simplify the radical expression.

\newline

\sqrt{12x^{12}}

\newline

Write your answer in the form

A

\sqrt{B}

A\sqrt{B}

A

B

are constants or expressions in

x

. Use at most one radical in your answer, and at most one absolute value symbol in your expression for

A

\newline

\underline{\hspace{3cm}}

Posted 2 months ago

Question

What is the value of

\sin \left(\frac{5 \pi}{6}\right) ?

\newline

1

\newline

-\frac{\sqrt{3}}{2}

\newline

-\frac{\sqrt{2}}{2}

\newline

\frac{1}{2}

\newline

150

Posted 4 months ago