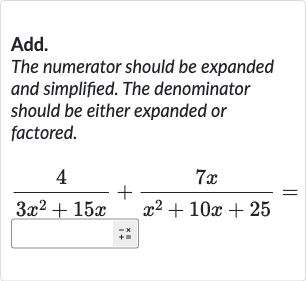
Add. The numerator should be expanded and simplified. The denominator should be either expanded or factored. (4)/(3x^(2)+15 x)+(7x)/(x^(2)+10 x+25)=

Question

Add. The numerator should be expanded and simplified. The denominator should be either expanded or factored.  (4)/(3x^(2)+15 x)+(7x)/(x^(2)+10 x+25)=

Bytelearn · Accepted Answer

Factor denominators: First, we need to factor the denominators of both fractions to see if they have common factors. The first denominator is 3x^2 + 15x. We can factor out a common factor of 3x: 3x^2 + 15x = 3x(x + 5). The second denominator is x^2 + 10x + 25. This is a perfect square trinomial: x^2 + 10x + 25 = (x + 5)^2.Rewrite expression with factored forms: Now that we have factored both denominators, we can rewrite the original expression with these factored forms: (4)/(3x(x + 5)) + (7x)/((x + 5)^2).Find common denominator: To add these fractions, they need to have a common denominator. The least common denominator (LCD) is 3x(x + 5)^2. We will adjust each fraction to have this common denominator.Adjust first fraction: The first fraction already has a factor of 3x(x + 5) in the denominator, so we need to multiply it by (x + 5) to get the LCD: (4)/(3x(x + 5)) * ((x + 5)/(x + 5)) = (4(x + 5))/(3x(x + 5)^2).Adjust second fraction: The second fraction has a denominator of (x + 5)^2, so we need to multiply it by 3x to get the LCD: (7x)/((x + 5)^2) * (3x/3x) = (21x^2)/(3x(x + 5)^2).Add fractions: Now we can add the two fractions since they have the same denominator: (4(x + 5))/(3x(x + 5)^2) + (21x^2)/(3x(x + 5)^2).Combine numerators: Combine the numerators over the common denominator: (4x + 20 + 21x^2)/(3x(x + 5)^2).Simplify numerator: Simplify the numerator by combining like terms: (21x^2 + 4x + 20)/(3x(x + 5)^2).Final simplified form: The numerator is already simplified, and the denominator is fully factored. There are no common factors to cancel, so this is the final simplified form of the expression.

Add. $\newline$ The numerator should be expanded and simplified. The denominator should be either expanded or factored. $\newline$ $\frac{4}{3 x^{2}+15 x}+\frac{7 x}{x^{2}+10 x+25}=$

Full solution

More problems from Factor sums and differences of cubes

Related topics

Add.\newlineThe numerator should be expanded and simplified. The denominator should be either expanded or factored.\newline43x2+15x+7xx2+10x+25= \frac{4}{3 x^{2}+15 x}+\frac{7 x}{x^{2}+10 x+25}= 3x2+15x4​+x2+10x+257x​=

Add. $\newline$ The numerator should be expanded and simplified. The denominator should be either expanded or factored. $\newline$ $\frac{4}{3 x^{2}+15 x}+\frac{7 x}{x^{2}+10 x+25}=$