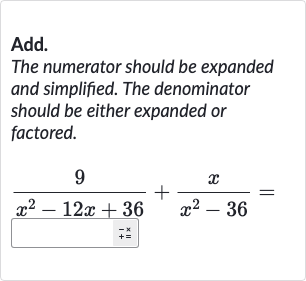
Add. The numerator should be expanded and simplified. The denominator should be either expanded or factored. (9)/(x^(2)-12 x+36)+(x)/(x^(2)-36)=

Question

Add. The numerator should be expanded and simplified. The denominator should be either expanded or factored.  (9)/(x^(2)-12 x+36)+(x)/(x^(2)-36)=

Bytelearn · Accepted Answer

Factor denominators: First, we need to factor the denominators of both fractions to see if they can be simplified or if there is a common denominator. The first denominator is x^2 - 12x + 36. This is a perfect square trinomial and can be factored as (x - 6)^2. The second denominator is x^2 - 36. This is a difference of squares and can be factored as (x + 6)(x - 6).Write fractions with factored denominators: Now that we have factored both denominators, we can write the fractions with their factored denominators: (9)/(x^2 - 12x + 36) becomes (9)/((x - 6)^2) (x)/(x^2 - 36) becomes (x)/((x + 6)(x - 6))Find common denominator: To add these fractions, we need a common denominator. The least common denominator (LCD) is (x - 6)^2 * (x + 6). We will rewrite each fraction with the LCD as the denominator.Rewrite fractions with common denominator: The first fraction already has (x - 6)^2 in the denominator, so it does not change: (9)/((x - 6)^2) The second fraction needs to be multiplied by (x - 6)/(x - 6) to have the LCD as the denominator: (x)/((x + 6)(x - 6)) * ((x - 6)/(x - 6)) = (x(x - 6))/((x - 6)^2 * (x + 6))Add fractions: Now we can add the two fractions with the common denominator: (9)/((x - 6)^2) + (x(x - 6))/((x - 6)^2 * (x + 6))Combine numerators: Combine the numerators over the common denominator: (9 + x(x - 6))/((x - 6)^2 * (x + 6))Expand and simplify numerator: Expand and simplify the numerator: 9 + x^2 - 6x = x^2 - 6x + 9Final simplified form: Now we have the simplified numerator over the common denominator: (x^2 - 6x + 9)/((x - 6)^2 * (x + 6))We can check if the numerator can be factored further, but it is already a perfect square trinomial, so it cannot be factored further. The final simplified form of the sum is: (x^2 - 6x + 9)/((x - 6)^2 * (x + 6))

Add. $\newline$ The numerator should be expanded and simplified. The denominator should be either expanded or factored. $\newline$ $\frac{9}{x^{2}-12 x+36}+\frac{x}{x^{2}-36}=$

Full solution

More problems from Factor sums and differences of cubes

Related topics

Add.\newlineThe numerator should be expanded and simplified. The denominator should be either expanded or factored.\newline9x2−12x+36+xx2−36= \frac{9}{x^{2}-12 x+36}+\frac{x}{x^{2}-36}= x2−12x+369​+x2−36x​=

Add. $\newline$ The numerator should be expanded and simplified. The denominator should be either expanded or factored. $\newline$ $\frac{9}{x^{2}-12 x+36}+\frac{x}{x^{2}-36}=$