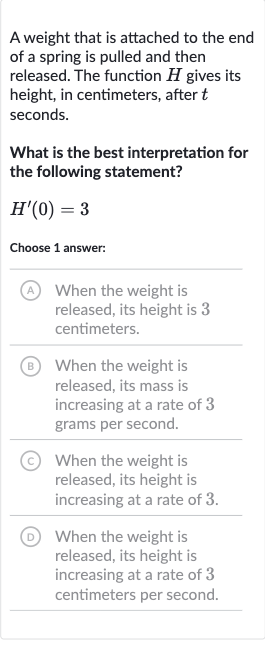
A weight that is attached to the end of a spring is pulled and then released. The function H gives its height, in centimeters, after t seconds. What is the best interpretation for the following statement? H^(')(0)=3 Choose 1 answer: (A) When the weight is released, its height is 3 centimeters. (B) When the weight is released, its mass is increasing at a rate of 3 grams per second. (c) When the weight is released, its height is increasing at a rate of 3 . (D) When the weight is released, its height is increasing at a rate of 3 centimeters per second.

Question

A weight that is attached to the end of a spring is pulled and then released. The function  H gives its height, in centimeters, after  t seconds. What is the best interpretation for the following statement?  H^(')(0)=3 Choose 1 answer: (A) When the weight is released, its height is 3 centimeters. (B) When the weight is released, its mass is increasing at a rate of 3 grams per second. (c) When the weight is released, its height is increasing at a rate of 3 . (D) When the weight is released, its height is increasing at a rate of 3 centimeters per second.

Bytelearn · Accepted Answer

Rate of Change Interpretation: H^(')(t) represents the rate of change of the height of the weight with respect to time. So, H^(')(0)=3 means that at time t=0, the rate of change of height is 3.Unit Conversion: Since the unit of height is given in centimeters and time is in seconds, the rate of change of height, H^(')(0)=3, is in centimeters per second.Height Increase Rate: The correct interpretation of H^(')(0)=3 is that when the weight is released, its height is increasing at a rate of 3 centimeters per second.

Full solution

More problems from Interpreting Linear Expressions

Related topics