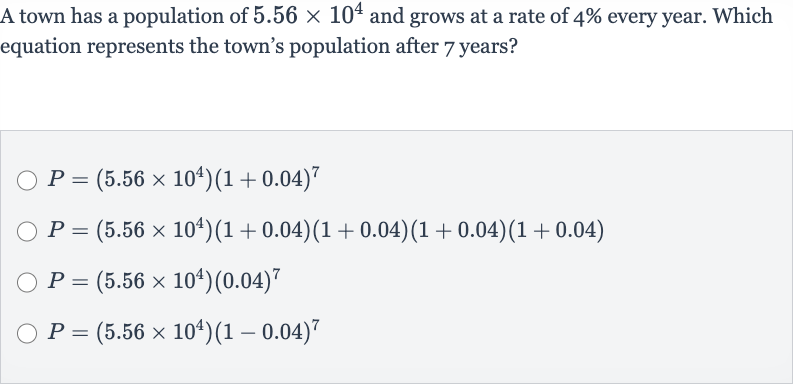A town has a population of $5.56 \times 10^{4}$ and grows at a rate of $4 \%$ every year. Which equation represents the town's population after $7$ years? $\newline$ $P=\left(5.56 \times 10^{4}\right)(1+0.04)^{7}$ $\newline$ $P=\left(5.56 \times 10^{4}\right)(1+0.04)(1+0.04)(1+0.04)(1+0.04)$ $\newline$ $P=\left(5.56 \times 10^{4}\right)(0.04)^{7}$ $\newline$ $P=\left(5.56 \times 10^{4}\right)(1-0.04)^{7}$

Full solution

Q. A town has a population of

5.56 \times 10^{4}

and grows at a rate of

4 \%

every year. Which equation represents the town's population after

7

years?

\newline

P=\left(5.56 \times 10^{4}\right)(1+0.04)^{7}

\newline

P=\left(5.56 \times 10^{4}\right)(1+0.04)(1+0.04)(1+0.04)(1+0.04)

\newline

P=\left(5.56 \times 10^{4}\right)(0.04)^{7}

\newline

P=\left(5.56 \times 10^{4}\right)(1-0.04)^{7}

Exponential Growth Formula: To find the population after $7$ years with a growth rate of $4\%$ per year, we use the formula for exponential growth: $P = P_0(1 + r)^t$ , where $P_0$ is the initial population, $r$ is the growth rate, and $t$ is the time in years.
Given Values: The initial population $P_0$ is given as $5.56 \times 10^4$ . The growth rate $r$ is $4\%$ , which is $0.04$ in decimal form. The time $t$ is $7$ years.
Substitute Values: Substitute the given values into the exponential growth formula: $P = (5.56 \times 10^4)(1 + 0.04)^7$ .
Correct Representation: The equation $P = (5.56 \times 10^4)(1 + 0.04)^7$ correctly represents the population after $7$ years, considering a $4\%$ annual growth rate compounded once per year.
Evaluation of Options: The other options can be evaluated for correctness: $\newline$ - $P = (5.56 \times 10^4)(1 + 0.04)(1 + 0.04)(1 + 0.04)(1 + 0.04)$ does not correctly apply the compound interest formula, as it only multiplies the growth factor $4$ times instead of $7$ . $\newline$ - $P = (5.56 \times 10^4)(0.04)^7$ incorrectly raises the growth rate to the power of $7$ instead of applying it as a percentage increase. $\newline$ - $P = (5.56 \times 10^4)(1 - 0.04)^7$ incorrectly assumes a decrease by $4\%$ per year instead of an increase.