Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

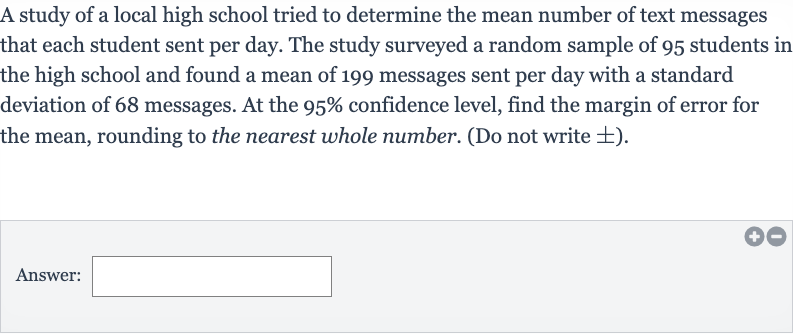
A study of a local high school tried to determine the mean number of text messages that each student sent per day. The study surveyed a random sample of 95 students in the high school and found a mean of 199 messages sent per day with a standard deviation of 68 messages. At the
95% confidence level, find the margin of error for the mean, rounding to the nearest whole number. (Do not write
+- ).
Answer:

A study of a local high school tried to determine the mean number of text messages that each student sent per day. The study surveyed a random sample of $95$ students in the high school and found a mean of $199$ messages sent per day with a standard deviation of $68$ messages. At the $95 \%$ confidence level, find the margin of error for the mean, rounding to the nearest whole number. (Do not write $\pm$ ). $\newline$ Answer:

View step-by-step help

View step-by-step help

Interpret confidence intervals for population means

Full solution

Q. A study of a local high school tried to determine the mean number of text messages that each student sent per day. The study surveyed a random sample of

95

students in the high school and found a mean of

199

messages sent per day with a standard deviation of

68

messages. At the

95 \%

confidence level, find the margin of error for the mean, rounding to the nearest whole number. (Do not write

\pm

).

\newline

Answer:

Identify Given Information: Identify the given information. $\newline$ We have a sample mean $\bar{x}$ of $199$ messages, a standard deviation $\sigma$ of $68$ messages, and a sample size $n$ of $95$ students. We want to calculate the margin of error at the $95\%$ confidence level.
Determine Critical Value: Determine the critical value (z-score) for the $95\%$ confidence level. $\newline$ For a $95\%$ confidence level, the z-score typically used is $1.96$ . This value is obtained from a standard normal distribution table or z-score table.
Calculate SEM: Calculate the standard error of the mean (SEM). The standard error of the mean is calculated using the formula $SEM = \frac{\sigma}{\sqrt{n}}$ , where $\sigma$ is the standard deviation and $n$ is the sample size. $SEM = \frac{68}{\sqrt{95}}$ $SEM \approx \frac{68}{9.7468}$ $SEM \approx 6.976$
Calculate Margin of Error: Calculate the margin of error. $\newline$ The margin of error ( $E$ ) is calculated using the formula $E = z \times SEM$ , where $z$ is the z-score and $SEM$ is the standard error of the mean. $\newline$ $E = 1.96 \times 6.976$ $\newline$ $E \approx 13.67296$
Round Margin of Error: Round the margin of error to the nearest whole number. $\newline$ Rounding $13.67296$ to the nearest whole number gives us $14$ .

More problems from Interpret confidence intervals for population means

Question

Michael surveyed

2

politicians from his state about their views on certain issues.

\newline

Is this sample of citizens of the state likely to be biased?

\newline

\newline

\newline

Posted 7 months ago

Question

Read the following description of an experiment.

\newline

A massage therapist has received complaints that the fragrance of her massage oil is unpleasant. She is testing whether a new oil will improve each clients' experience.

\newline

The massage therapist randomly sorts her clients into two groups. She massages

5

clients from the first group with the new oil and

5

from the other group with her usual oil. She asks all

10

clients to rate their massages.

\newline

Is this experiment statistically well designed?

\newline

\newline

\newline

Posted 7 months ago

Question

A random sample of

35

four-door passenger vehicles had a mean gas mileage, in miles per gallon

(\mathrm{mpg})

25.9 \mathrm{mpg}

. The estimate had a margin of error of

2.6 \mathrm{mpg}

98 \%

confidence level. Of the following, which is the most plausible value for the true mean gas mileage of all four-door passenger vehicles?

\newline

1

\newline

24 \mathrm{mpg}

\newline

29 \mathrm{mpg}

\newline

32 \mathrm{mpg}

\newline

35 \mathrm{mpg}

Posted 8 months ago

Question

1

578

randomly selected American adults,

44.8 \%

of the respondents said that airlines should allow in-flight calls on airplanes. The poll reported a margin of error of

2.5 \%

95 \%

confidence level. Which of the following is most likely to be equal to the percentage of all American adults who would say that airlines should allow in-flight calls?

\newline

1

\newline

40 \%

\newline

43 \%

\newline

48 \%

\newline

95 \%

Posted 10 months ago

Question

Malik walked up and back down an empty

18 \mathrm{~m}

long escalator at a constant rate. Since the escalator was going up at the time, it took him only

22.5 \mathrm{~s}

. Going down took Malik

90

s. Assume the escalator speed is constant.

\newline

What was Malik's walking speed?

\newline

\frac{\mathrm{m}}{\mathrm{s}}

Posted 8 months ago

Question

Two baseball players are warming up by throwing balls to the catcher. The two players are located

10

yards apart, and the angle from each player to the catcher is

55^{\circ}

71^{\circ}

, respectively. What is the distance from the farthest baseball player to the catcher?

\newline

10.125

\newline

11.687

\newline

14.501

\newline

14.790

Posted 8 months ago

Question

Select the outlier in the data set.

\newline

74,84,86,87,89,91,92,98,126

Posted 8 months ago

Question

p

\begin{array}{l}\frac{p}{8}=\frac{7}{9} \\p=\square\end{array}

Posted 8 months ago

Question

Jelani needs to hire at least

12

artists for an upcoming event. He needs to hire visual artists, who will be paid

\$300

for the event, and performers, who will be paid

\$480

for the event. His budget for paying the artists is no more than

\$6,000

. He must hire at least

4

visual artists and at least

3

performers. Which of the following does not satisfy all of the conditions described?

\newline

1

\newline

4

visual artists and

10

\newline

8

visual artists and

8

\newline

\$300

0

visual artists and

3

\newline

\$300

2

visual artists and

4

Posted 8 months ago

Question

\newline

k^2 + 9k + 18

\newline

\newline

Posted 8 months ago

Related topics

Algebra - Order of Operations Algebra - Distributive Property `X` and `Y` Axes Geometry - Scalene Triangle Common Multiple Geometry - Quadrant