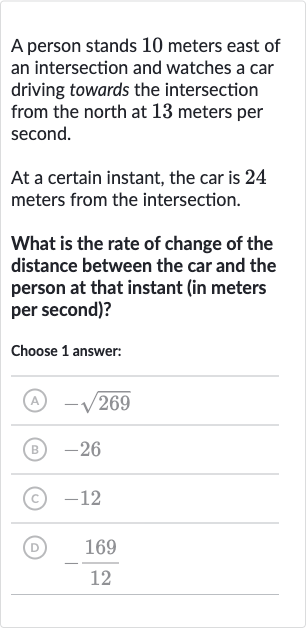
A person stands $10$ meters east of an intersection and watches a car driving towards the intersection from the north at $13$ meters per second. $\newline$ At a certain instant, the car is $24$ meters from the intersection. $\newline$ What is the rate of change of the distance between the car and the person at that instant (in meters per second)? $\newline$ Choose $1$ answer: $\newline$ (A) $-\sqrt{269}$ $\newline$ (B) $-26$ $\newline$ (C) $-12$ $\newline$ (D) $-\frac{169}{12}$

View step-by-step help

Home

Math Problems

Algebra 1

Rate of travel: word problems

Full solution

Q. A person stands

10

meters east of an intersection and watches a car driving towards the intersection from the north at

13

meters per second.

\newline

At a certain instant, the car is

24

meters from the intersection.

\newline

What is the rate of change of the distance between the car and the person at that instant (in meters per second)?

\newline

Choose

1

answer:

\newline

(A)

-\sqrt{269}

\newline

(B)

-26

\newline

(C)

-12

\newline

(D)

-\frac{169}{12}

Draw Triangle: First, let's draw a right triangle where the car is one vertex, the person is the second vertex, and the intersection is the third vertex. The car is $24$ meters from the intersection (north side), and the person is $10$ meters from the intersection (east side).
Find Hypotenuse: We need to find the hypotenuse of the triangle, which represents the distance between the car and the person at that instant. We use the Pythagorean theorem: $a^2 + b^2 = c^2$ , where $a$ is $24$ meters, $b$ is $10$ meters, and $c$ is the hypotenuse.
Calculate Hypotenuse: Calculating the hypotenuse: $c^2 = 24^2 + 10^2 = 576 + 100 = 676$ , so $c = \sqrt{676} = 26$ meters.
Find Rate of Change: Now, we need to find the rate of change of this distance. Since the car is moving towards the intersection, the distance between the car and the person is decreasing. We'll use related rates from calculus. Let's call the distance between the car and the person ' $z$ ', the distance of the car from the intersection ' $x$ ', and the distance of the person from the intersection ' $y$ '. We have $\frac{dz}{dt} = \left(\frac{dx}{dt} \cdot \frac{x}{z}\right) + \left(\frac{dy}{dt} \cdot \frac{y}{z}\right)$ . Since the person is not moving, $\frac{dy}{dt} = 0$ .
Use Related Rates: We know $\frac{dx}{dt} = -13$ meters per second (negative because the distance $x$ is decreasing), $x = 24$ meters, $y = 10$ meters, and $z = 26$ meters. Plugging these into the formula: $\frac{dz}{dt} = \left(-13 \times \frac{24}{26}\right) + \left(0 \times \frac{10}{26}\right)$ .
Apply Calculus: Simplifying the equation: $\frac{dz}{dt} = \frac{-312}{26} + 0 = -12$ meters per second. So, the rate of change of the distance between the car and the person is $-12$ meters per second.