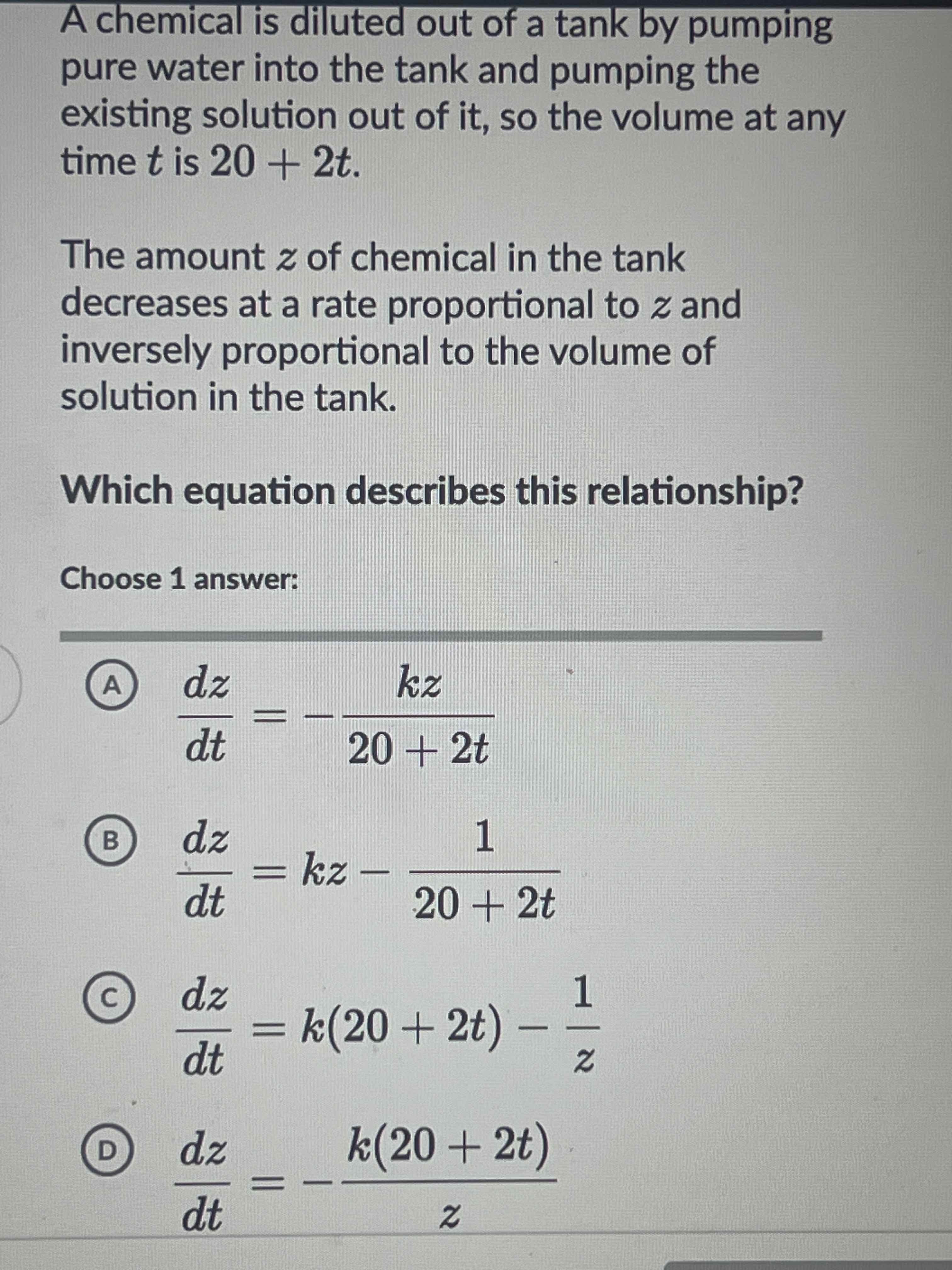
A chemical is diluted out of a tank by pumping pure water into the tank and pumping the existing solution out of it, so the volume at any time t is 20+2t. The amount z of chemical in the tank decreases at a rate proportional to z and inversely proportional to the volume of solution in the tank. Which equation describes this relationship? Choose 1 answer: (A) (dz)/(dt)=-(kz)/(20+2t) (B) (dz)/(dt)=kz-(1)/(20+2t) (c) (dz)/(dt)=k(20+2t)-(1)/(z) (D) (dz)/(dt)=-(k(20+2t))/(z)

Question

A chemical is diluted out of a tank by pumping pure water into the tank and pumping the existing solution out of it, so the volume at any time  t is  20+2t. The amount  z of chemical in the tank decreases at a rate proportional to  z and inversely proportional to the volume of solution in the tank. Which equation describes this relationship? Choose 1 answer: (A)  (dz)/(dt)=-(kz)/(20+2t) (B)  (dz)/(dt)=kz-(1)/(20+2t) (c)  (dz)/(dt)=k(20+2t)-(1)/(z) (D)  (dz)/(dt)=-(k(20+2t))/(z)

Bytelearn · Accepted Answer

Identify relationship:  Identify the relationship between the rate of change of the chemical amount and the volume of the solution. The rate of change of the chemical amount, $ \frac{dz}{dt} $, is proportional to the amount of chemical $ z $ and inversely proportional to the volume of the solution $ 20 + 2t $. Formulate equation:  Formulate the equation based on the relationship. Since $ \frac{dz}{dt} $ is proportional to $ z $ and inversely proportional to $ 20 + 2t $, the equation should be $ \frac{dz}{dt} = -\frac{kz}{20 + 2t} $, where $ k $ is a constant of proportionality. Match with options:  Match the formulated equation with the given options. The correct equation $ \frac{dz}{dt} = -\frac{kz}{20 + 2t} $ corresponds to option (A).

Full solution

More problems from Transformations of quadratic functions

Related topics