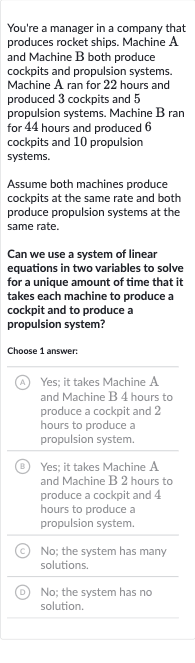
You're a manager in a company that produces rocket ships. Machine A and Machine B both produce cockpits and propulsion systems. Machine A ran for 22 hours and produced 3 cockpits and 5 propulsion systems. Machine B ran for 44 hours and produced 6 cockpits and 10 propulsion systems. Assume both machines produce cockpits at the same rate and both produce propulsion systems at the same rate. Can we use a system of linear equations in two variables to solve for a unique amount of time that it takes each machine to produce a cockpit and to produce a propulsion system? Choose 1 answer: (A) Yes; it takes Machine A and Machine B 4 hours to produce a cockpit and 2 hours to produce a propulsion system. (B) Yes; it takes Machine A and Machine B 2 hours to produce a cockpit and 4 hours to produce a propulsion system. (C) No; the system has many solutions. D No; the system has no solution.

Question

Bytelearn · Accepted Answer

Set Up Equations: Let's denote the time it takes Machine A to produce a cockpit as x hours and the time it takes to produce a propulsion system as y hours. Similarly, Machine B takes the same amount of time for each since they produce at the same rate. We can set up two equations based on the information given: For Machine A: 3x + 5y = 22 (since it produced 3 cockpits and 5 propulsion systems in 22 hours) For Machine B: 6x + 10y = 44 (since it produced 6 cockpits and 10 propulsion systems in 44 hours)Identify Relationship: We notice that the second equation is exactly double the first equation. This means that if we divide the second equation by 2, we will get the first equation: (6x + 10y) / 2 = 44 / 2 3x + 5y = 22 This indicates that the two equations are not independent; they are multiples of each other.Equations Represent Same Line: Since the two equations are not independent, they represent the same line in a coordinate system. Therefore, there are infinitely many solutions to this system of equations, as any point on the line that these equations represent is a solution.Infinite Solutions: Given that there are infinitely many solutions, we cannot determine a unique amount of time it takes for each machine to produce a cockpit or a propulsion system based on the information provided. The answer to the question is (C) No; the system has many solutions.

Full solution

More problems from Interpret parts of quadratic expressions: word problems

Related topics