Full solution
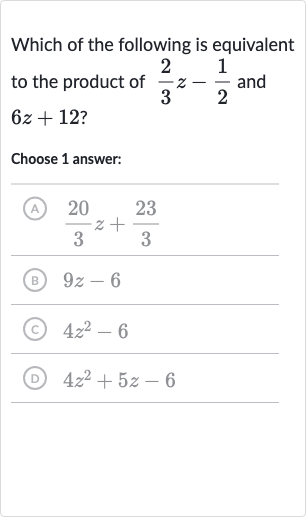
Q. Which of the following is equivalent to the product of and ?Choose answer:(A) (B) (C) (D)
- Multiply Expressions: We need to multiply the two given expressions: and . We will use the distributive property to do this, which states that . So we will multiply each term in the first expression by each term in the second expression.
- Multiply by : First, multiply by : .
- Multiply by : Next, multiply by : .
- Multiply by : Now, multiply by : .
- Multiply by : Finally, multiply by : (-\frac{\(1\)}{\(2\)}) \times \(12 = (\times/) = .
- Add Products: Add all the products together to get the final expression: .
- Combine Like Terms: Combine like terms: .
More problems from Identify equivalent linear expressions I
QuestionGet tutor help