Full solution
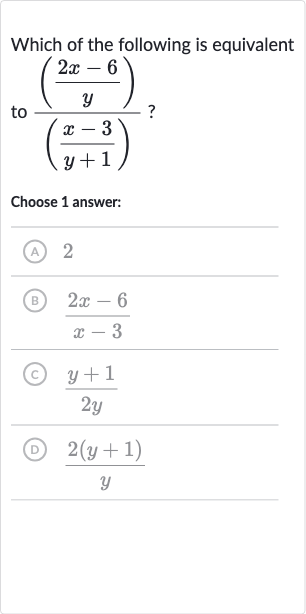
Q. Which of the following is equivalent to ?Choose answer:(A) (B) (C) (D)
- Simplify Expression: First, let's simplify the given expression by recognizing that dividing by a fraction is equivalent to multiplying by its reciprocal. The given expression is:To divide by a fraction, we multiply by its reciprocal. So, we rewrite the expression as:
- Factorize Numerator: Next, we notice that can be factored as . This simplification will help us see if there's any cancellation possible. So, the expression becomes:
- Cancel Terms: Now, we look for terms that can cancel out. We see that the terms in the numerator and denominator can cancel out, leaving us with:This simplifies to:
- Compare with Options: Finally, we compare the simplified expression to the given options. The expression matches option (D). Therefore, the equivalent expression is:
More problems from Identify equivalent linear expressions I
QuestionGet tutor help