Full solution
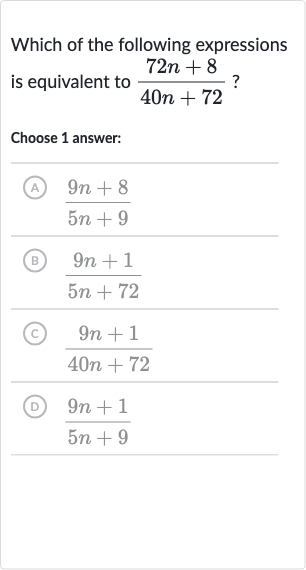
Q. Which of the following expressions is equivalent to ?Choose answer:(A) (B) (C) (D)
- Factor GCD: Factor out the greatest common divisor (GCD) from the numerator and the denominator.The GCD of and is , and the GCD of and is as well.So, we can factor out from both the numerator and the denominator.
- Simplify Expression: Simplify the expression by canceling out the common factor.Since is a common factor in both the numerator and the denominator, we can cancel it out.
- Compare with Options: Compare the simplified expression with the given options.The simplified expression matches with option (D).
More problems from Identify equivalent linear expressions I
QuestionGet tutor help