Full solution
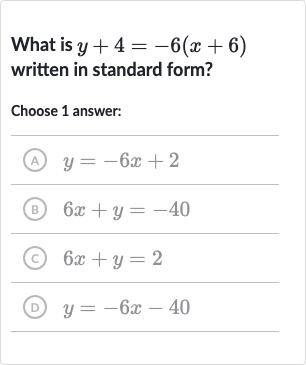
Q. What is written in standard form?Choose answer:(A) (B) (C) (D)
- Distribute across parentheses: First, we need to distribute the across the parentheses on the right side of the equation.
- Subtract from both sides: Next, we need to subtract from both sides to get the term by itself on the left side.
- Write equation in standard form: Now, we need to write the equation in standard form, which is , where , , and are integers, and should be non-negative.To do this, we add to both sides of the equation.
- Check for non-negative coefficient and integer terms: We check to make sure that the coefficient of is non-negative, which it is, and that all terms are integers, which they are. So the equation is now in standard form.
More problems from Evaluate rational expressions II
QuestionGet tutor help