AI tutor
Welcome to Bytelearn!
Let’s check out your problem:
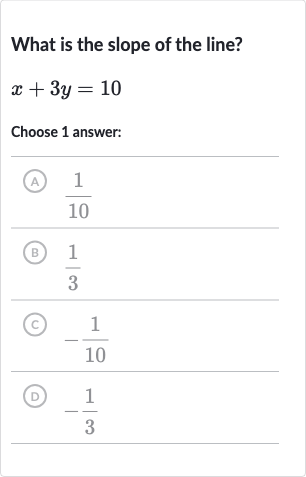
What is the slope of the line?Choose answer:(A) (B) (C) (D)
Full solution
Q. What is the slope of the line?Choose answer:(A) (B) (C) (D)
- Isolating the term with y: We start by subtracting from both sides of the equation to isolate the term with .This simplifies to:
- Solving for y: Next, we divide each term by to solve for .This simplifies to:
- Identifying the slope: Now that we have the equation in slope-intercept form, we can identify the slope. The coefficient of is the slope of the line. In this case, the coefficient of is .
- Identifying the slope: Now that we have the equation in slope-intercept form, we can identify the slope.The coefficient of is the slope of the line.In this case, the coefficient of is .Therefore, the slope of the line defined by the equation is .
