Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

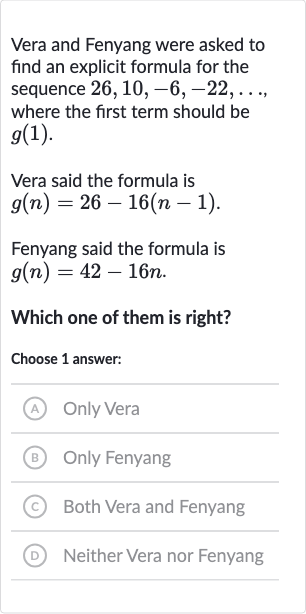
Vera and Fenyang were asked to find an explicit formula for the sequence 26,10,-6,-22,dots, where the first term should be g(1). Vera said the formula is g(n)=26-16(n-1). Fenyang said the formula is g(n)=42-16 n. Which one of them is right? Choose 1 answer: (A) Only Vera (B) Only Fenyang (C) Both Vera and Fenyang (D) Neither Vera nor Fenyang

Vera and Fenyang were asked to find an explicit formula for the sequence 26,10,−6,−22,… 26,10,-6,-22, \ldots , where the first term should be g(1) g(1) .\newlineVera said the formula is g(n)=26−16(n−1) g(n)=26-16(n-1) .\newlineFenyang said the formula is g(n)=42−16n g(n)=42-16 n .\newlineWhich one of them is right?\newlineChoose 11 answer:\newline(A) Only Vera\newline(B) Only Fenyang\newline(C) Both Vera and Fenyang\newline(D) Neither Vera nor Fenyang

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Algebra 2right chevron icon
Write a formula for an arithmetic sequenceright chevron icon

Full solution

Q. Vera and Fenyang were asked to find an explicit formula for the sequence 26,10,−6,−22,… 26,10,-6,-22, \ldots , where the first term should be g(1) g(1) .\newlineVera said the formula is g(n)=26−16(n−1) g(n)=26-16(n-1) .\newlineFenyang said the formula is g(n)=42−16n g(n)=42-16 n .\newlineWhich one of them is right?\newlineChoose 11 answer:\newline(A) Only Vera\newline(B) Only Fenyang\newline(C) Both Vera and Fenyang\newline(D) Neither Vera nor Fenyang
  1. Identify sequence type and common difference: Identify the type of sequence and the common difference.\newlineThe sequence given is 26,10,−6,−22,…26, 10, -6, -22, \ldots, which appears to be an arithmetic sequence because there is a constant difference between each term. To find the common difference, subtract the second term from the first term: 10−26=−1610 - 26 = -16. The common difference is −16-16.
  2. Test Vera's formula: Test Vera's formula.\newlineVera's formula is g(n)=26−16(n−1)g(n) = 26 - 16(n - 1). To check if Vera's formula is correct, substitute n=1n = 1 to find the first term: g(1)=26−16(1−1)=26−16(0)=26g(1) = 26 - 16(1 - 1) = 26 - 16(0) = 26. This matches the first term of the sequence, so Vera's formula is correct for the first term. Now, check the second term by substituting n=2n = 2: g(2)=26−16(2−1)=26−16=10g(2) = 26 - 16(2 - 1) = 26 - 16 = 10, which also matches the second term of the sequence. Vera's formula seems to be correct so far.
  3. Test Fenyang's formula: Test Fenyang's formula.\newlineFenyang's formula is g(n)=42−16ng(n) = 42 - 16n. To check if Fenyang's formula is correct, substitute n=1n = 1 to find the first term: g(1)=42−16(1)=42−16=26g(1) = 42 - 16(1) = 42 - 16 = 26. This matches the first term of the sequence. Now, check the second term by substituting n=2n = 2: g(2)=42−16(2)=42−32=10g(2) = 42 - 16(2) = 42 - 32 = 10, which also matches the second term of the sequence. Fenyang's formula seems to be correct so far.
  4. Determine correct formula: Determine which formula is correct.\newlineBoth Vera's and Fenyang's formulas have produced the correct first and second terms of the sequence. To determine which formula is correct, we need to check if the formulas will produce the correct terms for all positions nn in the sequence. Since both formulas have given the correct terms for n=1n = 1 and n=2n = 2, and both have the same common difference of −16-16, they will produce the correct terms for all nn. Therefore, both Vera and Fenyang are correct.

More problems from Write a formula for an arithmetic sequence

Question
Find the sum of the finite arithmetic series. ∑n=110(7n+4)\sum_{n=1}^{10} (7n+4)\newline______
Get tutor helpright-arrow

Posted 9 months ago

Question
Type the missing number in this sequence: `55,\59,\63,\text{_____},\71,\75,\79`
Get tutor helpright-arrow

Posted 5 months ago

Question
Type the missing number in this sequence: `2, 4 8`, ______,`32`
Get tutor helpright-arrow

Posted 5 months ago

Question
What kind of sequence is this? 2,10,50,250,…2, 10, 50, 250, \ldots Choices:Choices:\newline[A]arithmetic\text{[A]arithmetic}\newline[B]geometric\text{[B]geometric}\newline[C]both\text{[C]both}\newline[D]neither\text{[D]neither}
Get tutor helpright-arrow

Posted 5 months ago

Question
What is the missing number in this pattern? 1,4,9,16,25,36,49,64,81,____1, 4, 9, 16, 25, 36, 49, 64, 81, \_\_\_\_
Get tutor helpright-arrow

Posted 7 months ago

Question
Classify the series. ∑n=012(n+2)3 \sum_{n = 0}^{12} (n + 2)^3 \newlineChoices:\newline[A]arithmetic\text{[A]arithmetic}\newline[B]geometric\text{[B]geometric}\newline[C]both\text{[C]both}\newline[D]neither\text{[D]neither}
Get tutor helpright-arrow

Posted 5 months ago

Question
Find the first three partial sums of the series.\newline1+6+11+16+21+26+⋯1 + 6 + 11 + 16 + 21 + 26 + \cdots\newlineWrite your answers as integers or fractions in simplest form.\newlineS1=S_1 = ____\newlineS2=S_2 = ____\newlineS3=S_3 = ____
Get tutor helpright-arrow

Posted 7 months ago

Question
Find the third partial sum of the series. \newline3+9+15+21+27+33+⋯3 + 9 + 15 + 21 + 27 + 33 + \cdots\newlineWrite your answer as an integer or a fraction in simplest form. \newlineS3=S_3 = ____
Get tutor helpright-arrow

Posted 7 months ago

Question
Find the first three partial sums of the series. \newline1+7+13+19+25+31+⋯1 + 7 + 13 + 19 + 25 + 31 + \cdots\newlineWrite your answers as integers or fractions in simplest form. \newlineS1=S_1 = ____\newlineS2=S_2 = ____\newlineS3=S_3 = ____
Get tutor helpright-arrow

Posted 9 months ago

Question
Does the infinite geometric series converge or diverge?\newline1+34+916+2764+⋯1 + \frac{3}{4} + \frac{9}{16} + \frac{27}{64} + \cdots\newlineChoices:\newline[A]converge\text{[A]converge}\newline[B]diverge\text{[B]diverge}
Get tutor helpright-arrow

Posted 5 months ago

View all (93)

Related topics

Algebra - Order of OperationsAlgebra - Distributive Property`X` and `Y` AxesGeometry - Scalene TriangleCommon MultipleGeometry - Quadrant