AI tutor
Welcome to Bytelearn!
Let’s check out your problem:
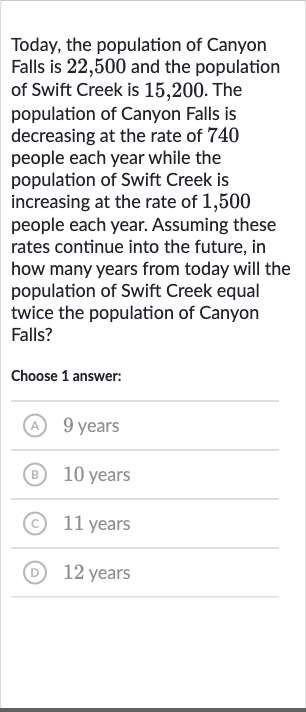
Today, the population of Canyon Falls is , and the population of Swift Creek is , . The population of Canyon Falls is decreasing at the rate of people each year while the population of Swift Creek is increasing at the rate of , people each year. Assuming these rates continue into the future, in how many years from today will the population of Swift Creek equal twice the population of Canyon Falls?Choose answer:(A) years(B) years(C) years(D) years
Full solution
Q. Today, the population of Canyon Falls is , and the population of Swift Creek is , . The population of Canyon Falls is decreasing at the rate of people each year while the population of Swift Creek is increasing at the rate of , people each year. Assuming these rates continue into the future, in how many years from today will the population of Swift Creek equal twice the population of Canyon Falls?Choose answer:(A) years(B) years(C) years(D) years
- Question prompt: Question prompt: In how many years from today will the population of Swift Creek equal twice the population of Canyon Falls, given their respective annual population change rates?
- Equation setup: Let's denote the number of years from today as . We need to set up an equation that represents the situation where the population of Swift Creek equals twice the population of Canyon Falls after years.
- Population of Canyon Falls: The population of Canyon Falls after years will be , since it decreases by people each year.
- Population of Swift Creek: The population of Swift Creek after years will be , since it increases by people each year.
- Equation solution: We want the population of Swift Creek to be twice the population of Canyon Falls. So, we set up the equation: .
- Solving for y: Now, we solve for "y". First, distribute the on the right side of the equation: .
- Final answer: Next, we combine like terms by adding to both sides and subtracting from both sides: .
- Final answer: Next, we combine like terms by adding to both sides and subtracting from both sides: . This simplifies to . Now, we divide both sides by to solve for : .
- Final answer: Next, we combine like terms by adding to both sides and subtracting from both sides: . This simplifies to . Now, we divide both sides by to solve for : . Performing the division gives us . Therefore, it will take years for the population of Swift Creek to equal twice the population of Canyon Falls.
