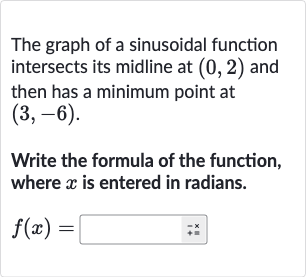
The graph of a sinusoidal function intersects its midline at (0,2) and then has a minimum point at (3,-6). Write the formula of the function, where x is entered in radians.

Question

The graph of a sinusoidal function intersects its midline at  (0,2) and then has a minimum point at  (3,-6). Write the formula of the function, where  x is entered in radians.

Bytelearn · Accepted Answer

Identify Amplitude, Midline, and Phase Shift: Identify the amplitude, midline, and phase shift of the sinusoidal function. The midline of the function is the horizontal line that the function oscillates around. Given the point (0,2), we know that the midline is y = 2. The minimum point of the function is (3,-6). Since the midline is y = 2 and the minimum value is -6, the amplitude of the function is the distance from the midline to the minimum, which is 2 - (-6) = 8. The function has a minimum at x = 3, which means the phase shift is 3 units to the right.Determine Period: Determine the period of the sinusoidal function. Since no information about the period is given, we assume the function has the same period as the basic sine function, which is 2π. Therefore, the period of the function is 2π.Write General Form: Write the general form of the sinusoidal function. The general form of a sinusoidal function is y = A * sin(B(x - C)) + D, where A is the amplitude, B is related to the period by the formula B = 2π / period, C is the phase shift, and D is the vertical shift (midline). We have already determined that A = 8, B = 1 (since the period is 2π), C = 3, and D = 2.Substitute Known Values: Substitute the known values into the general form of the sinusoidal function. Using the values A = 8, B = 1, C = 3, and D = 2, we substitute into the general form to get y = 8 * sin(x - 3) + 2. However, since the function has a minimum at x = 3, we need to use the cosine function, which starts at a maximum, and include a negative sign to reflect the graph vertically. This gives us y = -8 * cos(x - 3) + 2.

The graph of a sinusoidal function intersects its midline at $(0,2)$ and then has a minimum point at $(3,-6)$ . $\newline$ Write the formula of the function, where $x$ is entered in radians. $\newline$ $f(x)=\square$

Full solution

More problems from Write a quadratic function from its x-intercepts and another point

Related topics

The graph of a sinusoidal function intersects its midline at (0,2) (0,2) (0,2) and then has a minimum point at (3,−6) (3,-6) (3,−6).\newlineWrite the formula of the function, where x x x is entered in radians.\newlinef(x)=□ f(x)=\square f(x)=□

The graph of a sinusoidal function intersects its midline at $(0,2)$ and then has a minimum point at $(3,-6)$ . $\newline$ Write the formula of the function, where $x$ is entered in radians. $\newline$ $f(x)=\square$