The graph of a sinusoidal function intersects its midline at $(0,5)$ and then has a maximum point at $(0.75,7)$ . $\newline$ Write the formula of the function, where $x$ is entered in radians. $\newline$ $f(x)=\square$

View step-by-step help

Home

Math Problems

Algebra 2

Find trigonometric ratios using a Pythagorean or reciprocal identity

Full solution

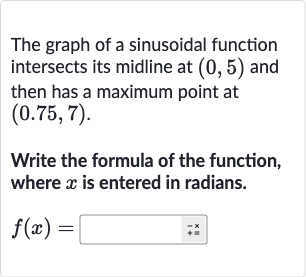
Q. The graph of a sinusoidal function intersects its midline at

(0,5)

and then has a maximum point at

(0.75,7)

\newline

Write the formula of the function, where

x

is entered in radians.

\newline

f(x)=\square

Determining Amplitude: The first step is to determine the amplitude of the sinusoidal function. The amplitude is the distance from the midline to the maximum or minimum point on the graph. Since the midline is at $y = 5$ and the maximum point is at $y = 7$ , the amplitude $A$ is $7 - 5 = 2$ .
Determining Period: The next step is to determine the period of the function. Since we are not given any information about the period, we will assume that the first maximum after the midline occurs at $x = 0.75$ . This means that $0.75$ is one-fourth of the period (since a sinusoidal function goes from midline to maximum to midline to minimum and back to midline in one full period). Therefore, the period $T$ is $0.75 \times 4 = 3$ .
Determining Phase Shift and Vertical Shift: Now we need to determine the phase shift and vertical shift. The phase shift is the horizontal shift from the standard position of the sinusoidal function. Since the function intersects the midline at $(0,5)$ , there is no horizontal shift, so the phase shift is $0$ . The vertical shift is the value of the midline, which is $5$ .
Writing the Sinusoidal Function: We can now write the formula for the sinusoidal function. Since the function reaches a maximum at $x = 0.75$ and not a minimum, we will use the sine function. The general formula for a sinusoidal function is $f(x) = A \cdot \sin(B(x - C)) + D$ , where $A$ is the amplitude, $B$ is the frequency (related to the period by $B = \frac{2\pi}{T}$ ), $C$ is the phase shift, and $D$ is the vertical shift. $\newline$ Plugging in the values $A = 2$ , $B = \frac{2\pi}{T} = \frac{2\pi}{3}$ , $C = 0$ , and $D = 5$ in $f(x) = A \cdot \sin(B(x - C)) + D$ .
Final Sinusoidal Function: $f(x) = 2 \times \sin\left(\frac{2\pi}{3}(x-0)\right) + 5$ $\newline$ The final formula for the sinusoidal function is: $\newline$ $f(x) = 2 \times \sin\left(\frac{2\pi}{3}x\right) + 5$