Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

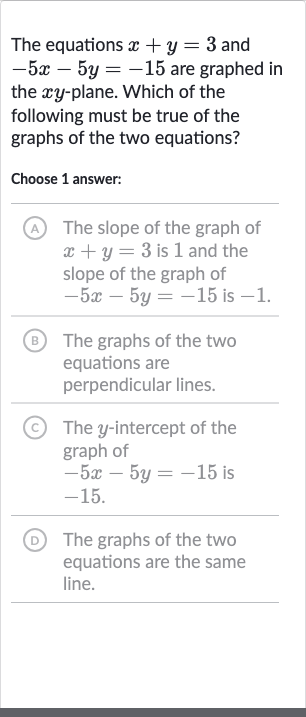
The equations x+y=3 and -5x-5y=-15 are graphed in the xy-plane. Which of the following must be true of the graphs of the two equations? Choose 1 answer: A The slope of the graph of x+y=3 is 1 and the slope of the graph of -5x-5y=-15 is -1 . (B) The graphs of the two equations are perpendicular lines. (C) The y-intercept of the graph of -5x-5y=-15 is -15 . (D) The graphs of the two equations are the same line.

The equations x+y=3 x+y=3 and −5x−5y=−15 -5 x-5 y=-15 are graphed in the xy x y -plane. Which of the following must be true of the graphs of the two equations?\newlineChoose 11 answer:\newline(A) The slope of the graph of x+y=3 x+y=3 is 11 and the slope of the graph of −5x−5y=−15 -5 x-5 y=-15 is −1-1 .\newline(B) The graphs of the two equations are perpendicular lines.\newline(C) The y y -intercept of the graph of −5x−5y=−15 -5 x-5 y=-15 is −15-15 .\newline(D) The graphs of the two equations are the same line.

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Algebra 1right chevron icon
Interpret parts of quadratic expressions: word problemsright chevron icon

Full solution

Q. The equations x+y=3 x+y=3 and −5x−5y=−15 -5 x-5 y=-15 are graphed in the xy x y -plane. Which of the following must be true of the graphs of the two equations?\newlineChoose 11 answer:\newline(A) The slope of the graph of x+y=3 x+y=3 is 11 and the slope of the graph of −5x−5y=−15 -5 x-5 y=-15 is −1-1 .\newline(B) The graphs of the two equations are perpendicular lines.\newline(C) The y y -intercept of the graph of −5x−5y=−15 -5 x-5 y=-15 is −15-15 .\newline(D) The graphs of the two equations are the same line.
  1. Analyze first equation: Let's analyze the first equation x+y=3x + y = 3.\newlineTo find the slope, we can rewrite the equation in slope-intercept form, which is y=mx+by = mx + b, where mm is the slope and bb is the y-intercept.\newlinex+y=3x + y = 3 can be rewritten as y=−x+3y = -x + 3.\newlineHere, the slope (mm) is −1-1 and the y-intercept (bb) is 33.
  2. Analyze second equation: Now let's analyze the second equation -5x - 5y = -15＂).\(\newlineFirst, we simplify the equation by dividing all terms by \$-5\) to get \(x + y = 3\).\(\newline\)This is the same equation as the first one, which means the two lines are not distinct; they are the same line.\(\newline\)Therefore, they have the same slope, which is \(-1\), and the same y-intercept, which is \(3\).
  3. Evaluate answer choices: Now let's evaluate the answer choices given the information we have:\(\newline\)(A) The slope of the graph of \(x+y=3\) is \(1\) and the slope of the graph of \(-5x-5y=-15\) is \(-1\). This is incorrect because both slopes are \(-1\).\(\newline\)(B) The graphs of the two equations are perpendicular lines. This is incorrect because the lines are the same, not perpendicular.\(\newline\)(C) The y-intercept of the graph of \(-5x-5y=-15\) is \(-15\). This is incorrect because the y-intercept is \(3\), not \(-15\).\(\newline\)(D) The graphs of the two equations are the same line. This is correct because both equations simplify to the same line, \(x + y = 3\).

More problems from Interpret parts of quadratic expressions: word problems

Question
Solve by completing the square.\newlinem2−10m−29=0m^2 - 10m - 29 = 0\newlineWrite your answers as integers, proper or improper fractions in simplest form, or decimals rounded to the nearest hundredth.\newline`m` = ____ or `m` = _____
Get tutor helpright-arrow

Posted 8 months ago

Question
Find g(x) g(x) , where g(x) g(x) is the translation 5 5 units up of f(x)=x2 f(x)=x^2 .\newlineWrite your answer in the form a(x–h)2+k a(x–h)^2+k , where a a , h h , and k k are integers.\newlineg(x)= g(x)= ____
Get tutor helpright-arrow

Posted 7 months ago

Question
What is the range of this quadratic function?\newliney=x2−4x+4y = x^2 - 4x + 4\newlineChoices:\newline{y∣y≥2}\left\{y \mid y \geq 2\right\}\newline{y∣y≤0}\left\{y \mid y \leq 0\right\}\newline{y∣y≥0}\left\{y \mid y \geq 0\right\}\newlineall real numbers\text{all real numbers}
Get tutor helpright-arrow

Posted 5 months ago

Question
Write the equation of the parabola that passes through the points (1,0)(1,0), (2,0)(2,0), and (3,–16)(3,\text{–}16). Write your answer in the form y=a(x–p)(x–q)y = a(x – p)(x – q), where aa, pp, and qq are integers, decimals, or simplified fractions.\newline______
Get tutor helpright-arrow

Posted 5 months ago

Question
Complete the square. Fill in the number that makes the polynomial a perfect-square quadratic.\newlinef2+8f+_____f^2 + 8f + \_\_\_\_\_
Get tutor helpright-arrow

Posted 5 months ago

Question
Solve for h h .\newlineh2+39h=0 h^2 + 39h = 0 \newline\newlineWrite each solution as an integer, proper fraction, or improper fraction in simplest form. If there are multiple solutions, separate them with commas.\newlineh= h = ____\newline
Get tutor helpright-arrow

Posted 5 months ago

Question
Write a quadratic function with zeros −9-9 and −7-7.\newlineWrite your answer using the variable xx and in standard form with a leading coefficient of 11.\newlinef(x)=_____f(x) = \_\_\_\_\_
Get tutor helpright-arrow

Posted 5 months ago

Question
Find the equation of the axis of symmetry for the parabola y=x2y = x^2. \newlineSimplify any numbers and write them as proper fractions, improper fractions, or integers.\newline‾\underline{\hspace{3cm}}
Get tutor helpright-arrow

Posted 4 months ago

Question
Find g(x)g(x), where g(x)g(x) is the translation 88 units up of f(x)=x2f(x) = x^2.\newlineWrite your answer in the form a(x–h)2+ka(x – h)^2 + k, where aa, hh, and kk are integers.\newlineg(x)=g(x) = ______\newline
Get tutor helpright-arrow

Posted 4 months ago

Question
Solve for xx.\newlinex2=1x^2 = 1\newline\newlineWrite your answer in simplified, rationalized form.\newlinex=x = ______ or x=x = ______\newline
Get tutor helpright-arrow

Posted 5 months ago

View all (145)

Related topics

Algebra - Order of OperationsAlgebra - Distributive Property`X` and `Y` AxesGeometry - Scalene TriangleCommon MultipleGeometry - Quadrant